One can be reasonably certain that when the title of an article includes the phrase “The Nature of Reality”, thought provoking words must surely lie ahead. But when that same title seems to inquire about a gentleman’s socks, coupled with an image of said gentleman’s socks which happen to be mismatched and reflect very loud colors , one might be moved in a direction which suggests the article is not of a serious nature. Perhaps even some sort of parody.
It is my hope that you will be pleasantly surprised with the subtle genius of Irish physicist [John Bell] and his use of socks, washing machines, and a little math to show how we can test one of quantum physic’s most fundamental properties. A property that does indeed reside in the very nature of the reality we are a part of. Few people can say they understand the Bell Inequality down to its most fundamental level. Give me a little of your time, and you will be counted among these few.
A Question That Changed Everything
First, we need a brief trek through history. Our story begins not very long ago, but in a galaxy very, very far away. A lonely electron exists in an unknown state in our distant galaxy. I say unknown because we can’t know. The galaxy, and hence the electron is several million light years away. [Einstein’s] special theory of relativity puts a limit on how fast information can travel, making it impossible for us to know anything about the current state of the electron. [Einstein] doesn’t know,  however, that our electron is special as well. It’s entangled. This means it has a quantum link to another electron…an electron that happens to be in our very laboratory. The property of entanglement says the two electrons must have opposite spin. So if I measure the spin of the electron in our lab, I can know the spin of the electron in the galaxy millions of light years away. Impossible!
however, that our electron is special as well. It’s entangled. This means it has a quantum link to another electron…an electron that happens to be in our very laboratory. The property of entanglement says the two electrons must have opposite spin. So if I measure the spin of the electron in our lab, I can know the spin of the electron in the galaxy millions of light years away. Impossible!
There is no way around it…quantum theory does allow for this mysterious property of our reality. [Einstein] said it violated his relativity theory and its cosmic speed limit. A theory that has been proven beyond any doubt to be correct. How can this be? Which theory is right? [Einstein] proposed an alternative theory that would satisfy the paradox. He used what are known as local hidden variables, which you can catch up on in my first discussion of Bartlmann’s Socks.
Basically, it says the spins of the two electrons are predetermined. But this is not compatible with a core principle of quantum theory, which says the spin of the electrons cannot be known until a measurement is made. And thus a great battle was born, with the victor earning the right to define the nature of reality. Unfortunately, [Einstein] and [Niels Bohr], who was leading the charge for quantum theory, would not live to see the end of the battle. Fast forward a few decades and you come to a point in history where [John Bell] puts an end to the argument with the mother of all weapons – a pair of socks.
Socks, Washing Machines and Hot Water
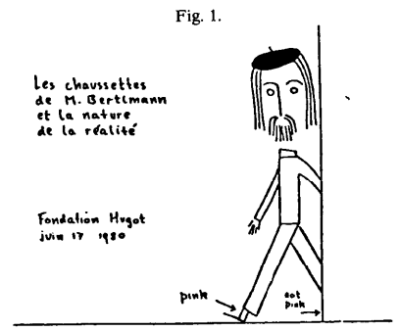 [Dr. Bertlmann] is known for wearing mis-matching socks to the lab. As his left foot enters through the door, you see that his sock is pink. Knowing what you know about Dr. Bertlmann], by observing the pink sock on one foot, you can derive information about the sock on the other. When you see a pink sock, you can say with absolute certainty that the other sock will not be pink.
[Dr. Bertlmann] is known for wearing mis-matching socks to the lab. As his left foot enters through the door, you see that his sock is pink. Knowing what you know about Dr. Bertlmann], by observing the pink sock on one foot, you can derive information about the sock on the other. When you see a pink sock, you can say with absolute certainty that the other sock will not be pink.
Let us borrow [Dr. Bertlmann’s] socks for some testing. We’re going to see how they hold up to prolonged washing at different temperatures. We’ll take the left socks and subject them to washing at three different temperatures. Let’s label the left socks “A” and the three temperatures “a“, “b” and “c“. We’ll wash each sock for the same length of time and then observe the results. Socks that are intact will be labeled “+” and socks that are destroyed will be labeled “–“. Note that for this experiment, the temperature of the water and the time of washing are not relevant.
Math-based Sock Washing Predictions
With a little math, we can derive some predictions of our experiment without actually have to carry through with it. Let us make set n(a+, b–) denote the socks that survived temperature a and were destroyed at temperature b. We can also write this value as the sum of two sub-sets. Let us imagine that a sock survives temperatures a and c, but gets destroyed at temperature b. We can write this as subset n(a+, b–, c+). Now take another sock that survives temperature a, but gets destroyed at temperatures’ b and c, which we represent with subset n(a+, b–, c–). We can say that either sock in these subsets must be a part of set n(a+, b–). The same can be done with set n(b+, c–), as this would be the sum of subsets n(a+, b+, c–) and n(a–, b+, c–). Now, let us set this up as an equality:
n(a+,b-) + n(b+,c-) = n(a+,b-,c+) + n(a+,b-,c-) + n(a+,b+,c-) + n(a-,b+,c-)

This headache inducing equation can be broken down as such: On the right side of the equal sign are the subsets and on the left are the sets. We went over how to get these subsets from the sets in the above paragraph. What we’re interested in is the two middle subsets. This is n(a+, b–, c–) + n(a+, b+, c–) which yields set n(a+, c–). How does this work from a math perspective? This is after all, an equality. You can’t fit n(a+, c–) on the left side of the equation. It doesn’t work. We can therefore conclude that:
The sum of sets n(a+, b–) and n(b+, c–) must be greater than or equal to set n(a+, c–).
Now, the observant reader will have realized that if a sock is destroyed at temperature a , it cannot be available for tests at temperature’s b or c. Let us not forget that [Dr. Bertlmann] had two feet, and socks come in pairs. They might be different colors, but their physical characteristics are considered to be the same. Such that the outcome of the tests on the left sock can be used to predict what the outcome will be for the right sock.
With access to pairs of socks and an understanding that the outcome of one half of the pair can be used to predict the outcome of the other, we can conduct a real experiment. We shall subject three samples of pairs of socks to three experiments. The left socks will retain their label of “A“, and the right socks will receive the label of “B“.
Experiment Time
We need to get around the problem of not being able to continue to test a sock because it was destroyed in a previous test. If a sock is destroyed at temperature a, how can we test it at temperature’s b or c? We’re going to get around this problem by using pairs. If I take a pair of socks, sock A and sock B, and subject each to different temperatures (or tests), I will get two results. Sock A will come out either intact (+) or destroyed (–) and sock B will come out similarly. Because the socks have the same physical characteristics, I can use the outcome of one test to predict the outcome in the other. What do I mean by that? If sock A is destroyed by temperature a, then I can make a prediction that sock B will also be destroyed at temperature a. This solves our dilemma, because if a sock is destroyed at temperature a, I can substitute in its pair for the following test. We can now proceed with a real world experiment.
Consider the chart below.
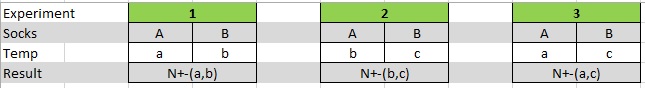
As you see, each experiment will test each of our socks at two different temperatures. For Experiment One, sock A will be subjected to temperature a and sock B will be subjected to temperature b. Then we will focus on the number of pairs of socks for which sock A survives (labeled ‘+’) and sock B is destroyed (labeled ‘-‘), which will be denoted as set N+-(a,b). The next point is key to understanding why we’re doing the test in this fashion.
During the hypothetical test, we realized that set n(a+, b–), which is the sum of subsets n(a+, b–, c+) and n(a+, b–, c–), cannot be accurately tested because socks destroyed at temperature b will not be available for test at temperature c. In our real world experiment, we will substitute in the other half of the pair when this occurs. By doing this, we can say that the number N+-(a,b) must be equal to our hypothetical number n(a+, b–).
For Experiment Two, we will wash sock A at temperature b and sock B and temperature c. We apply the same logic as above to deduce that N+-(b,c) is equal to n(b+, c–). The third and final experiment will put sock A at temperature a and sock B and temperature c and we conclude that the resulting set N+-(a,c) is equal to n(a+,c–).
The law of substitution tells us that the sum of sets N+-(a,b) and N+-(b,c) must be greater than or equal to set N+-(a,c). If we apply our logic in a generalized form as a sum of probabilities, we get:
P+-(a,b) + P+-(b,c) ≥ P+-(a,c)
This, ladies and gentlemen, is Bell’s Inequality. Take a look back at part one, and replace pairs of socks with entangled atoms, washing machines with magnets and temperatures with magnet orientations, and you have the same thing – the Bell Inequality. Let’s run some numbers so you can see what all the fuss is about.
If we pick up from where we left off at the end of part one, quantum theory says that P+-(a,b) = 1/2 cos2 (x/2) where x = (b – a). We can choose any angle for the magnets we want. Let’s say that:
Magnet A = 0°
Magnet B = 146°
Magnet C = 282°
Applying these values to the inequality would yield:
1/2 cos2 (73) + 1/2 cos2 (68) ≥ 1/2 cos2 (141)
or
11.2% ≥ 30.1%
Conclusion
The numbers are obvious. The predictions from quantum theory violate the Bell inequality. There is no getting around it. Remember, the angles of the magnets are represented as the temperature of the wash water. It doesn’t matter at what temperature we wash our socks, the sum of sets N+-(a,b) and N+-(b,c) must be greater than or equal to set N+-(a,c). Likewise, the same can be said for the angles of the magnets and atomic spin. From part one you learned how we use the angle of the magnets to determine if the spin of the atom is up (+) or down (–), which is analogous to a sock that survives or is destroyed. By changing the angle of the magnets and looking at probabilities for different spin outcomes, we can subject the paradox to an actual, measurable experiment. [John Bell] has given us the ability to perform a real world test to see who is right – [Einstein] and his hidden variables or [Bohr] and his quantum theory.
“If the hidden variable extension is local it will not agree with quantum mechanics, and if it agrees with quantum mechanics it will not be local.”
John Bell
When [Bell] put forth his inequality (pdf) to the scientific community on July, 18th 1980, the technology to actually carry out such a test was not yet in reach. But it was close. In 1982, French physicist [Alain Aspect] made it his thesis to carry out such a test. While the results leaned toward the direction of quantum theory, there were still loopholes that kept [Einstein’s] hidden variables on life support. But as technology caught up, the loopholes were eventually closed, giving quantum theory the winning hand.
The next time you see a person wearing mismatched socks, I hope you think about [John Bell] and how he used such a trivial part of our daily lives to prove a not so trivial part of our daily existence.
Sources:
The Quantum Story, by Jim Baggott. Chapter 31 ISBN-978-0199566846

















That was interesting, and provides a fragment of understanding to one aspect of the quantum puzzle.
I now need to look up how electrons become entangled and how this topic affects other realms of thought.
“I now need to look up how electrons become entangled ”
Entanglement isn’t special. Any time an electron is *created*, it’s entangled, because its properties (energy, position, momentum, spin) are coupled to all of the other particles that were created (or affected) at the same time, since you can’t just “poof” create an electron with a magic wand.
I mean, this is actually the way that particle physics is done. You measure information about every single particle that comes out of a collision, and then go back and reconstruct the original particle’s properties. So *obviously* every one of those particles is entangled. You measure one electron’s momentum, you measure the momentum of some other particle jet, add them together… and find out that way more often than you’d expect randomly, they add up to some specific value. Poof! The corresponding energy was the energy of that unstable particle. The momentum of those decay products were entangled.
So the better question might be ‘how do electrons become unentangled?” And the answer to that is “they interact with things.” All of the entangled electron measurements, all of the quantum crypotography stuff – they reason they’re all very hard to do is that you have to essentially make sure that the electrons aren’t interacting with *anything else* – or if they are, they’re doing it in such a way that the spin state is undisturbed. Mess with the electrons randomly at all, and all of that correlation goes away. This is actually how quantum cryptography works.
From a quantum mechanics point of view, this leads to understanding how “spooky action-at-a-distance” works. It works because distance doesn’t mean anything. The particles didn’t interact before they got to you. So for them, nothing’s happened, no time has passed, and they’re still back at the original thing that decayed.
That interpretation is taking the “local-real-causal” triad of Einstein and removing the “local” bit.
It leads to the problem that since your particles essentially exist “everywhere”, filling all space so you could claim they exist both separate at the two detectors and united in the original pair or particle, you’re claiming that their probability density functions exist through all space.
And that leads to the problem of collapsing the wavefunction, because it must happen throughout the entire universe instantly, which still means that distant part of the universe gain information about every other part of the universe faster than light.
Another interpretation which doesn’t break Einstein’s relativity, or causality, is to reject “realism”. That is to say, the properties being measured aren’t real – they only exist as temporary combinations of something else, like two sticks crossed make a cross, which has certain physical properties and behaviour as long as the configuration remains, and none of the properties when the sticks are again separated.
That means, the electron doesn’t have a spin except in combination with the measuring device, and so the properties of the measuring device affect what the spin turns out to be – and those properties are affected by information reaching it from the point in time and space when the electron pair was separated. In fact, the information about the event can reach the measuring device before the electron does.
That is to say, if we have two experiments running to detect the two electrons of an entangled pair, the information about the pair separating in the first place reaches the two spatially separated experiments at the speed of light, whereas the electrons themselves arrive slower than the speed of light, and so both experiments are “primed” to interact with the electrons in a way that creates correlated spins.
“It leads to the problem that since your particles essentially exist “everywhere””
Yeah. It’s almost like you’re saying that the particles are just excitation quanta of a field that exists everywhere.
Oh wait. They are.
Hm, that didn’t come out as clear as it did in my head. :)
What I mean is that you’re saying “consider that spin isn’t real.” I’m saying no, that doesn’t fix everything. It might fix this somehow, but quantum mechanics and relativity have lots of other problems (the Aharonov-Bohm effect, for instance, implies that a global EM 4-field isn’t a ‘real’ thing).
What fixes everything is that the *electron* as a whole isn’t real. It’s just a name that we give to a field excitation. And there’s no way to actually interact with those fields directly, except through the excitations themselves. So a spin-0 state creates 2 oscillations of the field, with coupled polarization, which go off and propagate.
“What fixes everything is that the *electron* as a whole isn’t real.”
That’s just the next step in the logic.
The same works with photons as well, when you think that the photon particle doesn’t exist, only the wavefunction of the photon exists.
So you have two wavefunctions A and B that start to spread in space and time from the separation event. One starts ever so slightly to the left, and another ever so slightly to the right as they are created from their mutual origin.
So anyone observing the event at the left would see these two wavefunctions arriving in the order AB, and anyone standing to the right would observe them arriving in the order BA, and while they’re both of the opinion that they’ve recieved a single photon each, they have actually recieved a composite of two wavefunctions, AB and BA, which result in what appears to be photons with complementary properties such as polarity.
The earlier arrival of the wavefunction that started closer to the observer primes the resulting observation of a photon so that even if the polarity or any other property being measured is random, it is still correlated with the other observer who recieves the same information but in opposite order.
Saying that the “collapse” of the mathematical description we call the wave function is a real event is probably the source of part of the problem. If in order to get an answer that fits experiment you have to integrate over all space, it doesn’t mean the electron can be anywhere in space.
And you have to look at spooky action “faster than light” in terms of the electrons involved. The electrons didn’t go faster than light. They are separated at some sub-light speed. This implies that the information about spin is communicated through the absolute elsewhere. But since you can’t measure it twice, you can’t know if this is true. Of course, if it IS instantaneous you have to wonder about extra collapsed dimensions and three generations of physicists wasted on String Theory. Also about macro evidence: Wouldn’t you expect some other universe wide instantaneous phenomena? And wouldn’t it be detectable?
Interesting stuff. One might think QM in the long run is a dead end if it didn’t predict results so brilliantly (but that was true of classical physics for a long time).
“And you have to look at spooky action “faster than light” in terms of the electrons involved. The electrons didn’t go faster than light. They are separated at some sub-light speed. This implies that the information about spin is communicated through the absolute elsewhere.”
No, it means that non-relativistic quantum mechanics is stupid. Which is what Einstein was trying to say. The problem is that his idea to replace it was even stupider. It’s still stupid, and it still needed to be replaced. And it was.
“One might think QM in the long run is a dead end if it didn’t predict results so brilliantly”
Non-relativistic quantum mechanics had a ton of problems, for obvious reasons – it totally doesn’t work when particles are moving close to the speed of light. Not at all. And physicists knew this. They just couldn’t get the “relativistic” part to work at all, because the math just didn’t work. And it still doesn’t work. The past 50 years have just been physicists getting better and better and better at quietly approximating a theory with broken math.
Thus the generations of bright people wasted on String Theory. For example look what Michio Kaku does these days. He is now a “futurist and popularizer of science”. String theory is a bust for physics, but a boon for fresh physicists seeking tenure I guess. It is what the ever more complicated Atwood’s Machines and their Hamiltonians were for classical physics.
As I understand it, the electrons become entangled because two electrons cannot occur in pairs and have the same spin. Pauli exclusion principle – it would be like having two magnets south-to-south – they flip around – literally, as the spins of the electrons are basically their magnetic orientations.
When you break up such a pair – assuming they’re not interacting with anything else – they retain their complementary spin orientations.
That is, if you shoot two electrons head on, they will assume opposite spins upon bouncing off one another. When you trace their paths in an external magnetic field, one should curve up and the other will curve down in your spin detector. All that seems really simple, and should result in the straight-line probability curve of the previous article when two spin-detectors are rotated in respect to one another.
The problem is that according to QM and practical experiment, the spin of the particle is essentially random – it’s a probability distribution. When the particle hits the detector, the probability of which direction it takes may be skewed by the earlier collision, but is not determined by it. As such, we shouldn’t be able to know what the other particle is doing based upon measuring the first, because they should both be independently random.
The entaglement bit comes in because the outcome of the collapse of the probability distribution of one particle is mirrored by the other particle across space and time as if it knew what the first particle was doing, without there being no possible way to exchange information between the particles.
So Einstein’s objection was that “God doesn’t play dice”, and that what seems like a random probability distribution is in fact driven by some local hidden variable that gives a deterministic history to both particles, which allows them to “know” one another without exchanging information after the collision. Think of it like the spins of the particles were being wiggled around by a pseudo-random number generator in each particle, that got initialized with the same seed number when the particles impacted one another so they each repeat the same sequence in a mirrored fashion. Bell then went to show that there is no possible “variable” like that.
“As I understand it, the electrons become entangled because two electrons cannot occur in pairs and have the same spin.”
No, that’s not it. You can create 2 electrons with the same spin direction. That’s easy. You just need a vector (spin 1) state to begin with. Pauli exclusion only applies if they’re completely confined to the same state. If they’re free electrons, there’s a continuum of states for them to be in.
The electrons become entangled because 2 electrons, together, have more phase space available in their spins than the original state had. So the 2 spins have to be coupled.
To follow the entire logic from the beginning, you start with a spin-0 state. It somehow produces 2 spin-1/2 particles. Normally those spins could be aligned in any direction. You could encode information in the relative angle between the two spins if you felt like it! But the original state was spin-0: *it had no information to pass along.* So the phase space of the 2-particle system isn’t fully populated, and you say “they’re entangled.”
But this is just another way of saying that you didn’t create a full “2 free electron” system.
” So the 2 spins have to be coupled.”
Arguing that they’re coupled and probabilistic results in Einstein’s “spooky action at a distance”, because messing with the spin of one essentially creates the spin of the other regardless of distance, which must happen faster than the speed of light. It rejects the idea of localism – that particles exist somewhere instead of everywhere or nowhere.
Rejecting localism or causality essentially breaks the laws of physics and/or our ability to have any meaningful theory of anything, so the only sensible thing left to do is to say that the two electrons don’t have spins until measured. The property that is entangled with another particle isn’t a real property, but instead an artifact of the measuring event.
That may or may not extend to the entire electron itself.
“Arguing that they’re coupled and probabilistic results in Einstein’s “spooky action at a distance”, because messing with the spin of one essentially creates the spin of the other regardless of distance,”
When I said “coupled spin” I didn’t mean they have 2 spins that are somehow linked. I meant they’re both produced with a correlation in the spin phase space of the 2 particle system. This is just shorthand. Following the logic, you’d say:
Experimenter 1: I believe I have a free electron, so I will measure its spin in some direction. Ah! I find that direction is “up.”
Experimenter 2: I believe I have a free electron, so I will measure its spin in some direction 5 degrees off from Experimenter 1. Ah! I find that direction is “down to me.”
Experimenter 1 and 2 come together: Hm. If we *really had* 2 free electrons, our spin measurements would be uncorrelated. But they are correlated. We did not really have 2 free electrons. We had a coupled 2-electron system. We were not actually measuring spins of a free particle.
In the “Bertlmann’s socks” example, it would be like what happened in the entangled state is that someone gave you something that you *thought* was a sock, but it wasn’t a sock, and you couldn’t tell the difference until you looked at the results from both socks combined.
So if I have a pair AB and I then separate them and hit A with C of a known spin, what happens to the state of B? What if there is also a pair CD and I hit A with C, what does that tell us about how B and D will interact? I ask because all this AB stuff is fine, but things get useful when you have AB CD EF etc.. then you can start computing and communicating with them.
“So if I have a pair AB and I then separate them and hit A with C of a known spin, what happens to the state of B? ”
Nothing. Nothing *happens* to the state of B at all. You just got some information about the combined (A,B) state. It’s exactly the same as if you have a particle decay into 2 others. You go off and search for particle A, and then as soon as you find particle A… you know where particle B is. So it’s exactly like if you have 2 researchers, one searching on one side, and one searching on another side. If they’re searching by themselves, they might conclude “oh my goodness, as soon as I find mine, the other one teleports directly to the location where my colleague is!” If they were crazy, that is.
Obviously nothing’s teleporting. Now, naively, you might say “that’s just silly – the other particle was *always* going to be at that spot.” And that’s where Bell’s theorem comes in – spin gives you a simple way to show that “no, you can’t say that. It can’t have been predetermined.”
“What if there is also a pair CD and I hit A with C, what does that tell us about how B and D will interact?”
You start to build up the basics of a quantum computer.
“I ask because all this AB stuff is fine, but things get useful when you have AB CD EF etc.. then you can start computing and communicating with them.”
Computing, yes, communicating, no. There’s no information passed between entangled states. You’re not influencing the other one. It’s correlation, not causation.
But when C, which has a known state because we read D, interacts with B it imposes a known, opposite to B, state on A, it is knowable because D and B can’t have the same state as each other when they interact. Combine that with a choice of being able to discard C if D does not have the value we wish to communicate and you get the ability to flip the state of remote A in a conditional way, make it reflect a local state. So what part is broken there, because it does see so simple it would have been done if it worked at all. It is that the state of C can’t force B, and therefore A, to resolve to the opposite of D?
[A(1)…..{B(0)] [C(1)},D(0)]
I know the nesting of the brackets is weird there but so is the overlapping states of multiple pairs, it is that weirdness that suggests that one can selectively control the, ….. remote, state of A() by conditionally allowing the {} multi-pair interaction.
Is the gotcha in the laws of physics, or in what we currently know about controlling overlapping states of multiple pairs?
“But when C, which has a known state because we read D, interacts with B it imposes a known, opposite to B, state on A”
Nope. C interacting with B does nothing to A. It can’t do anything to it.
B and A aren’t “linked” in any way: it’s not like if you wiggle B, A wiggles too. No. They just share information – they’re 2 objects that have the *capability* of storing 2 bits of information, but they actually only store 1. Now, you can use ‘B’ to figure out what A *will* do. But you can’t actually *do* anything to A.
” It can’t do anything to it.”
So you say, but why can’t it? This is the key knowledge I am seeking.
What specifically prevents the (A{B)(C}D) super-entangled state from forming? I would have thought that it can form but that the influence works both ways negating the benefit, or in reading D you collapse the entire “quantum chain” and that is why it can’t work as an data link, but I don’t know what known law proves this is the case. Why can’t chains exist of any length? (A{B)(C}[D)(E]{F)(G}H)…
Why can’t the chains be virtual particle chain interactions, and why isn’t this how the effect is propagating in the first place?
What if I have two forms of entanglement, or three and I am able to generate pairs so that there is a coherence between the types of entanglement within the particles so that when I measure Aa it implies something about Ab and Ac without me having to read Ab and Ac directly, therefore giving me access to knowledge about Bb and Bc.
I do accept that “you probably can’t do it because nobody is doing it” as a strong indication that it may not be possible, but I don’t understand the mechanism that enforces this situation.
“So you say, but why can’t it? This is the key knowledge I am seeking.”
Because you only interacted with B. Why would anything happen to A?
“What specifically prevents the (A{B)(C}D) super-entangled state from forming?”
I think you’re getting caught up on the idea/word of “entangled state.” Entanglement doesn’t mean there’s anything *causal* that connects them. It’s just a correlation between them. It’s another way of saying “these two electrons share some information.” Or “these 2 electrons aren’t *actually* free electrons, but we want to pretend they are, so we call them ‘entangled free electrons’ to represent the phase space they’re *missing*.”
Shine a flashlight at the moon. .Wave your hand in front of it. On the surface of the moon, that means that a shadow is moving across the surface of the moon faster than the speed of light! So what? It doesn’t do anything. There’s no causal connection between the photons that arrive on one side of the moon to the other side. It’s just a corrrelation.
“Why can’t the chains be virtual particle chain interactions, and why isn’t this how the effect is propagating in the first place?”
There’s no effect that’s propagating at all. It’s just information. The Universe encoded the fact that it was a spin-0 original state into 2 particles (A and B), and those particles went on their way. If you interact with B in such a way that it depends on the spin, then the resulting state (B’) no longer has that encoded information. So let’s say you have a second particle (C), and you interact B with C. So now C becomes C’, and maybe that information got transmitted to C’. So now (A, C’) are correlated. But (A, B’) are uncorrelated.
You can do cool things with this idea, but none of them are FTL communication, because nothing’s being communicated. For instance, if (A, B) are correlated, and (C, D) are correlated, you can make a measure on (B,C) and communicate those results to A/D, resulting in an entanglement of A/D. This is called “entanglement swapping”.
But from an information basis, you can see what’s going on here: there’s a bit (e.g. a spin component) of information between (A,B), and a bit of information between (C,D), so 2 total bits. And so you extract the difference between those bits from (B,C) – which gives you 1 bit – and communicate them to A and D, so they can get the remaining bit.
So FTC is only blocked by the fact that we can’t do this, make (A-PropertyType1-[B)-PropertyType2-{C]-PropertyType2-D} links such that we can be sure that we can sort the super groups so that the state of D is correlated with A, even though A and D do not directly share an entangled property? i.e. we can’t join the dice (and only use the ones that where the group are coherent [111] vs [101] or [110] etc) so that when we roll them we can know the result on A by observing D to determine the outcome of observing A before we do so, and that means that we cannot then discard the option to observe B if it would cause the unwanted result in the remote A? The laws of physics do not stop us from cheating, the fact that we can’t both load the dice and also know which ones will suit us, that is the barrier?
11.2% ≥ 30.1% Is this supposed to demonstrate a greater than? Eleven percent is not greater than Thirty percent. Is that what demonstrates that Bell inequality is wrong?
It’s proof by contradiction – it’s basically showing that from these premises follows a statement that is not true, such as 1 = 0 or 1 > 2 therefore one or the other assumptions must be false.
Yes. In part one, I go over how to make a simple hidden variables theory. Bell figured out that in any type of such theory, his inequality would exist. Quantum mechanics produces numbers that violate this inequality. It gives a way to test which theory is true.
Careful!
It gives a way to exclude one of the theories, which it does – it excludes a hidden-variable interpretation of that system. It doesn’t show that the quantum’s version is true, and in fact, physicists really *like* locality, so the common interpretation of Bell’s theorem (“our universe really is nonlocal, and relativity is wrong!”) is *not* what theorists did. Instead, they went the way that Dax and myself were trying to say above, which is that they abandoned the idea that “an electron” is this ‘real’ thing that you can always measure.
There’s actually four outs from the Bell inequalities.
Non-locality, non-reality, non-causality and the fourth is superdeterminism, which is a kind of “Goddidit”, that events aren’t happening randomly, probabilistically, nor following one another deterministically, but simply unfolding like the pages of a book or frames of a film reel, where the contents of one page or frame aren’t caused by nor result in any other, yet they don’t exist randomly.
Such a universe might be like reading the number Pi from start to infinity, and at some point finding a detailed description of our universe and its evolution through time and space. It doesn’t actually happen – it just is.
For those interested, Eliezer Yudkowsky’s Intuitive Explanation of Quantum Mechanics also includes an article on how this doesn’t violate Special Relativity, even without hidden variables: Spooky Action at a Distance: The No-Communication Theorem.
This is your last chance. After this, there is no turning back. You take the blue sock – the story ends, you wake up in your bed and believe whatever you want to believe. You take the red sock – you stay in Wonderland and I show you how deep the rabbit-hole goes.
I was hoping to see this.
Why would entangled particles lead to any kind of faster-than-light data tranfser? By observing the particle in the lab and inferring the state of the particle a million light years away, you aren’t getting any data from the far away particle, you’re just reading a copy of the information. The copy was stored in your lab. Nothing special or magic or quantum-y is going on. Or am I oversimplifying?
“Why would entangled particles lead to any kind of faster-than-light data tranfser?”
They don’t. You’re not oversimplifying. You’re completely right.
The whole idea of “quantum mechanics is magic!” really just persists from all of these gedanken experiments that people came up with at the turn of the century because quantum mechanics was new and only Kinda Sorta Worked. Nowadays we think of quantum mechanics as being super-awesome and exact, but in the 1920s-30s, it was a total hack. So people tried to come up with crazy thought experiments to explain how it was a hack, because, well, it totally *looked* like a hack.
In the end we actually did figure out “this non-relativistic thing really is a hack.” Unfortunately, all of the crazy thought experiments stuck around, and so you if you think about things in 1930s-level physics knowledge, things look really weird.
Because by QM each of the particles arriving at you don’t have pre-determined states. They are probabilistic entities which “collapse” into a state when you measure them.
It’s as if someone threw a randomly spinning coin at you. When you grab it from the air and put it on your hand, you lock it to heads or tails. The weirdness comes from the fact that there was two coins thrown in the air, and another person grabbed the other coin, and when you repeat the coin toss he always gets the opposite result from yours because the two coins are “entangled”, even though the coins are supposed to be spinning randomly.
The non-realist explaination of this is that both were actually thrown a blank coin, and the act of grabbing it from the air turns your coin into “heads” and the other person’s coin into “tails” because you’re primed to do that by earlier interactions with the coin-tosser.
The non-local explanation of this is that both coins extend in space to their point of origin, so that you two are in fact holding the same coin and looking at the opposite sides of it.
The non-causal explaination of it says that the outcome had nothing to do with the coin toss, or the coins, and the opposite results, or the information that you have obtained opposite results, only occurs when you and the other person come together to compare results.
The Bell inequalities assert that at least one of the three, locality, realism, causality, must be abandoned to explain the results.
About your coin example –
I throw two coins at the same time from my hand.
Because I have thrown them at the same time, they have the same “spin” or tumble rate as it were, but they are traveling in slightly different paths.
Now both you and your friend grab their respective coin at the same time and in the same way.
When you both look at your coins, they are opposite. We repeat this experiment and see the same opposite face result.
Why is it not possible that the electrons behave much like the coins where the face you get is always random, but your friend always gets the opposite face? I must be missing something.
“Because I have thrown them at the same time, they have the same “spin” or tumble rate as it were, but they are traveling in slightly different paths.”
That is a hidden variable theory.
QM posits that the “coins” aren’t tumbling at any rate, but that their orientation is actually indetermined, that is, they’re facing every which way at once. They are not face up or face down at different parts of the journey, but face up AND face down all the way to you (superposition of states), both individually as long as we stick to the idea that they are separate coins (localism) that exist completely (realism). You simply grab one state out of the superposition and it becomes the result for all future events (causality)
That means the two coins aren’t doing equal but opposite flips through the air. They’re both neither up nor down, and so grabbing one means that information would have to somehow travel to the other coin to say “Hey, I got caught, you need to assume the opposite state”, because collapsing one superposition collapses the other.
Can you explain to me why this sends information, faster than the speed of light (or actually send information at all).
I get the part that we could call the collapse (that your coin is “locked” in state after i grab mine) “sending information”, but I don’t think any information is actually transmitted. There is no way for me to know, that you grabbed the coin, when I grabbed mine and got a tails (and vice versa). So no information can be transmitted in this way. If i somehow force a heads on my end, I would suspect that it break the entanglement, without forcing your coin into a tails.
“Can you explain to me why this sends information, faster than the speed of light (or actually send information at all).”
By the fact that one coin feels the collapse of the superposition of the other. Something goes over instantly, faster than light, across any distance, also into the past and the future because each coin’s superposition is supposed to break only at the moment when the coin is caught – not before or after – because there’s nothing around that would interact with it before you catch it. In some moving reference frames, both catchers could see themselves catching it first because of relativity. So the entanglement connects not only distance, but also time.
That is, if we insist on holding on to the local-real-causal triad. It doesn’t have to mean that you can use the coins to communicate – just that the coins necessarily communicate with each other. That is how Einstein and his followers used the argument to insist that reality is not probabilistic because it leads to this weirdness, so QM is simply wrong and there must be some hidden variable theory explaining it deterministically.
And you cannot force the coin to land any way, because the moment you interact with it you break the superposition. When the superposition goes, the entanglement goes, and flipping the coin around no longer affects the other.
I didn’t read the whole article – quite lengthy, but you said “quantum theory does allow for this mysterious property of our reality”. When Einstein said Information can’t travel faster than light, he was careful in his word selection. Data can travel faster than light. You cannot communicate faster than light using entangled particles. It would be awesome, but as far as I have researched not possible among Scientific facts.
Why the hell not?!
Why can’t we manually entangle 2 strings of electrons, then put one set on a satellite, keep the other in a lab and use our set to communicate back and forth.
Come on physicist, get off your lazy butts and make it work!
[/sarcasm]
A “common sense” question for the peanut gallery. “If” all this theory about opposite spins, action-at-a-distance, yadda, yadda, yadda is true – what is the implication on a higher level (ie. actual material objects). Does anyone recall a sci fi movie called “Journey To The Far Side Of The Sun” ? Astronaut returns to Earth, but everything is opposite.. left is right, etc.
I agree. What implications does this all have? It’s interesting and all, but I don’t really care that much about it. Maybe I’d feel differently if I could see some application of this knowledge.
It’s the entire basis of quantum cryptography?
Quantum cryptography works because what’s going on here is that you’re actually encoding information into two separate objects in such a way that it’s completely impossible to probe the system while preserving that information.
In the “Bertlmann’s socks” analogy, what’s actually happening is that you thought you had 2 socks, and they were always the same color. You don’t. You actually have 2 pictures of the same sock, but these are amazing pictures: they self-destruct the instant you look at them. Which, if you think about it, is *exactly* what you want for secure communications.