Every high school physics student knows c, or the speed of light, it’s 3 x 10^8 metres per second. More advanced or more curious students will know that this is an approximation, and the figure of 299,792,458 metres per second that forms the officially accepted figure comes from a resonance of the caesium atom from which is derived a value for the second.

But for those who are really curious about measuring the speed of light the question remains: Just how did we arrive at that figure and how long have we been measuring it? The answer contains some surprises, and some exceptionally clever scientific thought and experimentation over the centuries.
The nature of light and whether it had a speed at all had been puzzling philosophers and scientists since antiquity, but the first experiments performed in an attempt to measure it were you will not be surprised to hear, performed by Galileo sometime in the early 17th century. His experiment involved his observation of assistants uncovering lanterns at known distances away, and his observations failed to arrive at a figure.
Later that century in 1676 the first numerical estimate of the speed of light was made by the Danish astronomer Ole Rømer, who observed an apparent variation in the period of one of Jupiter’s moons depending upon whether the Earth was approaching it or moving away from it. From this he was able to estimate the time taken for light to cross the Earth’s orbit, and from there the mathematician Christiaan Huygens was able to produce a figure of 220,000,000 metres per second.
Spinning Cogs And Mirrors: Time Of Flight
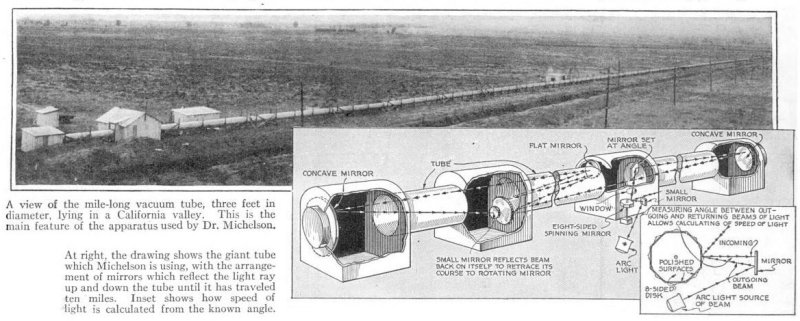
The experiments with which we will perhaps be the most familiar are the so-called time of flight measurements, which take Galileo’s idea of observing the delay as light travels over a distance, and bring to it ever higher precision. This was first performed in the middle of the 19th century by the French physicist Hippolyte Fizeau, who reflected a beam of light from a mirror over several kilometres, and used a toothed wheel to chop it into pulses. The pulses could be increased in frequency by moving the wheel faster until the time taken for the light to travel the distance from wheel to mirror and back again matched the separation between teeth and the returning pulse could be observed. His calculation of 313,300,000 metres per second was successively improved upon through the work of succession of others including Léon Foucault, culminating in the series of experiments by the American physicist Albert A. Michelson in the 1920s. Michelson’s final figure stood at 299,774,000 metres per second, measured through a multi-path traversal of a mile-long evacuated tube in the California desert. In the second half of the century the techniques shifted to laser interferometry, and in the quest to define the SI units in terms of constants, eventually to the definition mentioned in the first paragraph.
The most fascinating part of the story probably encapsulates the essence of scientific discovery, namely that while to arrive at something takes the work of many scientists building on the work of each other, it can then often be rendered into a form that can be understood by a student who hasn’t had to pass through all that effort. We could replicate Fizeau and Michelson’s experiments with a pulse generator, laser diode, and oscilloscope, which while of little scientific value nearly a century after Michelson’s evacuated tube, is still immensely cool. Has anyone out there given it a try?
Header image: Tommology, CC BY-SA 4.0.














This was demonstrated (the Fizeau method) by AlphaPhoenix on Youtube. He shows that it can be done with very basic equipment.
http://www.youtube.com/watch?v=YMO9uUsjXaI
You want to follow A great thought experiment? Consider the question : What if c is not a constant? Then follow that both directions – What if its speeding up? what if its slowing down? Wrote a fascin, to me, paper during my doctoral research on one of those ideas and found A very old text ,thought by most to be legend, could be amazingly accurate.
“How Did We Get To The Speed Of Light?”
Asymptotically.
😂
This is the winner!
Did I type that?
by being bright 🌞 😂
I know of one recent attempt to reproduce the Fizeau experiment: https://www.youtube.com/watch?v=YMO9uUsjXaI
I remember reading someplace that nobody has yet conceive a way of measuring the speed of light directly–that is to say, in one direction. In fact, such a measurement may be physically impossible because of Relativity and other factors.
I gather that all measurement schemes involve a beam of light that is sent in one direction and then returned, a closed loop, if you will.
You measure the total time of flight–outbound plus inbound–and then divide by two. This is because it is assumed that the speed of light in the outbound direction will be the same as the speed of light in the inbound direction.
But that is only an assumption!
That leads to the intriguing possibility of hidden asymmetry… that light could travel in one direction instantaneously, for example, and then return at the speed of c/2.
Light is impacted by gravity, therefore, from the bottom of a gravity well. It will be blue shifted coming in and red shifted coming out. Time also slows with increase in the G field. So the two are inextricably linked. What is you measurement frame of reference.
Veritasium covered it: https://www.youtube.com/watch?v=pTn6Ewhb27k
>That leads to the intriguing possibility of hidden asymmetry
There is the principle of necessary effect. If something is truly hidden, then it doesn’t even exist in this reality because it makes no difference. To debate there is or isn’t such asymmetry when it couldn’t possibly be measured would be nonsense.
Many physics thought experiments fail on this very point. For example, Schrödinger’s Cat. If you have a box which perfectly isolates an object from observation, placing an object there would effectively erase it from the universe and constitute a violation of the conservation energy and information. Practically speaking, the universe has to “feel” that something is there for it to be there. If it doesn’t then you can’t say that a cat will come back out when you open the box.
Also, if the conservation laws are maintained after all, trapping the Schrödinger’s Cat in the box would cause the largest gamma-ray burst made by man as the entire mass-energy of the cat turns into light just as the lid is closing.
You could weaponize it or turn it into a very efficient propulsion system by adding a hole with a shutter on the side of the box.
“For example, Schrödinger’s Cat. If you have a box which perfectly isolates an object from observation, placing an object there would effectively erase it from the universe and constitute a violation of the conservation energy and information.”
Nope. The box needs to effectively shield all information transfer (all worldlines) from *inside* to *outside*. Sound familiar? It’s a black hole.
This is actually in some sense the underpinning of Penrose’s idea that gravity functionally limits quantum entanglement. Because you can’t shield gravity, period, at some point it becomes impossible to maintain perfect (macroscopic) entanglement because gravity disturbs it.
Hmm, isn’t this hitting the black hole information paradox?
The photon sphere of a black hole, where centrifugal force goes to zero, might be a place where you could measure the single-directional speed of a photon, but there are a lot of issues with setting up experimental apparatus there.
How about Time Domain Reflectometry (TDR)?
It’s a technique by which pulses with a very steep rise-time can be seen on an oscilloscope, traveling to some discontinuity (open, start, or other change in characteristic impedance), and then being reflected back to the source.
It’s not light, per se, but even modest oscilloscopes are capable of doing it, with even modest (nanosecond) pulse generators.
Fibre optic engineers do optical TDR every day, it’s been a thing for decades.
I’ve seen that video on YouTube and he’s wrong. No need for kilometers of distance with fast electronics. Texas Instruments TDC7200 has a resolution of 55 spec. A pulsed laser diode as a source, A beam splitter to get a START signal near the laser and a detector a few meters away to get a STOP signal. The START and STOP signals to the the TDC though equal length cables off to the side and voila! A one way time measurement. Mikroe has a breakout board with the TI chip on it (https://www.mikroe.com/tdc-click). Add that to a microprocessor of your choice and you’ve got it. Maybe less than $100. If you already have most of the stuff, the TDC is only $15.
Psec not spec!!!!
psec != Psec
Yeah the video covers that, and exactly why it wont work.
And the reason is, if I understand correctly, that the signals coming from start and stop sensors in the equal length cables go in opposite direction, so it is again the average speed of the round trip that is measured.
The signals don’t go in opposite directions though… one is coming back, that’s for sure, the other would depend on how the experiment lays out the nearby cable, it could be a circle, in which case you’d notice a difference if there’s a difference to notice by sending the far signal in different directions.
I’m not sure I’m visualizing correctly what you are describing, but if you mean to roll one cable into a spiral to keep the first cable near the first tag, it doesn’t work either. The signal in the rolled up cable will just constantly change it’s orientation and the overall speed will still average any anisotropy. You could maybe imagine what happens if the axis of rotation of the spiral is colinear to the measured light beam. Then you could check if the speed is the same in the axis of the beam or in the perpendicular axis but I think it would still compare the 2 way speeds in perpendicular directions.
No, still not getting it. We’re on a table top, not kilometers of distance between the START and STOP. And the cables from the START and STOP are at Right Angels to the direction of the light beam. There is no Coming Back here. Use logic, not what some guy on YT says.
And if that doesn’t convince you, then you can invest in expensive Time Taggers (~$7000). One at the START and one at the STOP. They record the absolute time down to 2 psec, using GPS, Subtract the two times and you have the time; you know the distance from measuring it with a ruler. If Einstein could use clocks and rulers to come up with relativity then we can do the same to measure the one way speed of light. 55 psec is a little over a centimeter. 2 psec is about 0.3 mm With that kind of resolution it only takes a few meters to do the experiment.
And why would I believe some guy on Hackaday over these people?
—
The script was written in consultation with subject matter experts:
Prof. Geraint Lewis, University of Sydney https://ve42.co/gfl
Prof. Emeritus Allen Janis, University of Pittsburgh
Prof. Clifford M. Will, University of Florida https://ve42.co/cmw
—
You can’t compare the timing of events “here” and “there” according to relativity. It’s all, well, relative to the observer. All you can do is measure when something happened “there” from the perspective of the “here” observer, and that is subject to whatever the “speed of causality” is on the path from “there” to “here”. You know nothing about the perspective of the “there” observer. All you can do is average the “here” to “there” plus “there” to “here” path.
I don’t see how the fact that you are on a smaller scale would change anything to the logic. Just that you need more precise equipement to mesure the time. Sync by GPS doesn’t work because the signal coming from the satellite arrive from different directions to both time tags if the satellite is equidistant to the tags. If it isn’t equidistant then they aren’t synced properly to check this. Therefore you need to Sync the tags at the same place then move one of them which will unsync them. Not trying to sound pedant here, I’m just trying to use logic and not what some guy on HaD says.
Scale is irrelevant
Every high school physics student knows c, or the speed of light, it’s 3 x 10^8 metres per second.
How much is that in freedom units?
“How much is that in freedom units?” 186,262 miles per second. Everyone knows that.
A foot per nanosecond (+/-10%) everyone knows that.
I remember a video of Grace Hopper using an ~11 inch cable as a prop in a lecture. Of course I suspect that wasn’t taking account of the actual speed of an electric signal in a wire, but it was still informative. I always thought about that when I first saw pictures of a Cray-1 with all he interconnect wires running in the interior “hole” to minimize length.
They were ‘nanoseconds’.
I recall them being shorter…about 10-15 cm. Which would be about the correct distance, assuming you approximate voltage propagation in wire at 1/2 C. 30cm is a nanosecond in vacuum.
Cray signal wires were tuned to all arrive at the same time. Every year or so, they dove in with scopes and trimmed wires to account for components changing properties. Your phone is faster.
They were 30 cm according to what I could find. She later started using pepper grains to represent picoseconds.
“Cray signal wires were tuned to all arrive at the same time.”
True. But making them as short as possible by routing them through the center also made it faster. The Cray-1 ran at 80MHz, giving you 12.5 ns per clock. So 12 ft-ish at light speed… and as you say, about half that in a wire. And then add in transistor switching time…
“Your phone is faster.”
Absolutely. There’s a guy that implemented a Cray-1 in an FPGA.
I knew it as 186,282 miles per second LONG before I knew the metric value. :-)
It’s easy to remember that sound travels at ~300m/s and light travels at 300m m/s. Not precise, but close enough for any mental arithmetic.
Outstanding math.
6 x 10^10 ft/min
(The “which units” debate is silly, BTW.)
“I got pulled over by the cops for doing the speed of light in a speed of sound zone. The cop asked me if I heard his siren… I told him I didn’t even see his lights…” – Mork from Ork (Mork and Mindy.. paraphrased).. AR R R R R..
Na-no na-no
Old, OLD joke … A scientist was pulled over for going through a red signal. At court, he explained to the judge that the Doppler effect had changed the red light to appear green. The judge asked how fast he would need to drive for this effect. After the scientist answered, the judge changed the infraction to speeding.
There’s another angle to this story about how certain these scientists were about their estimates — essentially, how much we know about what we know (or don’t know). Psychologists Max Henrion and Baruch Fischhoff looked at the estimates and confidence intervals for experiments on the speed of light from 1875 to 1958. How good are we at knowing what we know? Not very good. Until about 1950, the now-accepted true value of c fell outside the 95% confidence interval for most estimates. Something I try to remember every time I’m certain I’m right.
https://www.researchgate.net/profile/Baruch-Fischhoff/publication/244419115_Assessing_Uncertainty_in_Physical_Constants/links/56afbdc708ae9ea7c3ad9e51/Assessing-Uncertainty-in-Physical-Constants.pdf
Don’t forget, you can use peeps, a ruler, and a microwave to find the speed of light.
Forgot link
https://youtu.be/HwREvdUWSKE
That video has an awesome explainer of the Fizeau setup. With marshmallows. Animated. Winner!
The melt-in-microwave “measurement” leaves me a little cold though. How do I know the frequency of the microwave? It’s not measurable in the same way that a spinning disk is — i.e. with gear reductions and pens on paper, which is the way Fizeau did it.
But melting stuff in the microwave is an awesome demo of standing waves. No doubt.
I’m still trying to think up a clever photodiode and cheap laser pointer version of the Foucault spinning mirror version. Something you could do wtih an Arduino and the internal timers, for instance.
I suppose you could remove the spinning tray and put a neon bulb in the microwave and accurately measure the node/antinode spacing by its brightness.
Many many years ago I worked in a lab that had a very fast time interval measuring device. (Nanofast?) I was amazed that by setting up a light source and two identical sensors one foot apart and both aimed at the light source, I could measure the time difference as the light source was turned on or off. (One sensor started the timer the second one turned it off).
Just as much so, what were the flaws in the process of calculating it?
I read a story once about a guy troubleshooting for a company. The problem was that their email would only go… About 500 miles. Any farther and it would fail.
It turned out to be a problem with the time to live settings. 500 miles was apparently ratherclose to the distance light should cover in the time it was set to.