[Math the World] claims that your calculus teacher taught you integration wrong. That’s assuming, of course, you learned integration at all, and if you haven’t forgotten it. The premise is that most people think of performing an integral as finding the area under a curve or as the “antiderivative.” However, fewer people think of integration as adding up many small parts. The video asserts that studies show that students who don’t understand the third definition have difficulty applying integration to real-world problems.
We aren’t sure that’s true. People who write software have probably looked at numerical integration like Simpson’s rule or the midpoint rule. That makes it pretty obvious that integration is summing up small bits of something. However, you usually learn that very early, so you’re forgiven if you didn’t get the significance of it at the time.
Even if you didn’t learn calculus, the video is an easy introduction to the idea of integration with practical examples drawn from basketball, archery, and more. Although there is a bit of calculus terminology, the actual problems could just as easily have been the voltage on a charging capacitor, for example.
We think calculus has a bad rap as being difficult when it isn’t. Maybe you should take more than 20 minutes to learn it.

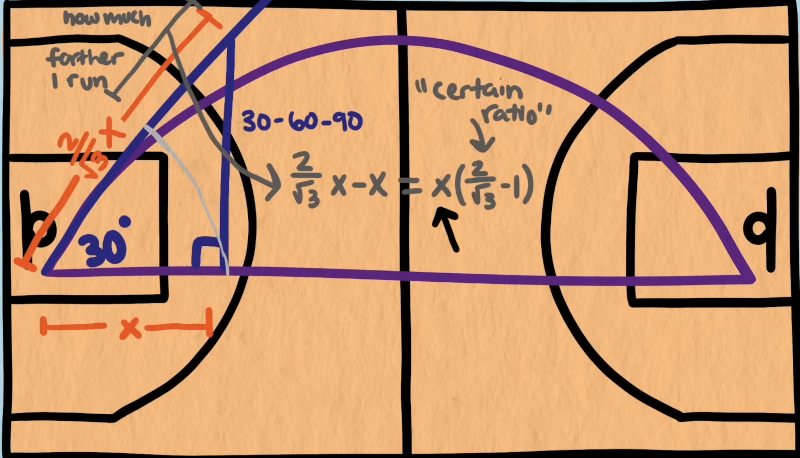














Doesn’t do much good if my goldfish brain can’t remember all the formulas regarding the integration of sin and cos and others
https://youtu.be/nNb6EpHhqTM?si=jr_FLuBq3xJwuEAh
I still have this memorized from my high school calc teacher.
Eh, maybe an unpopular opinion but I think memorizing integration formulas is overrated outside of a basic calculus course. Sure it’s something you probably have to do to learn perhaps, but there’s not a ton of practical value in memorizing these. I’m a full time scientist and have a BS in Math and a PhD in a related field and I practically never do any integration by hand, nor does anybody I work with. If I actually need to work out an integral by hand and not just in the abstract, either I can do it by eye (add one to the power and divide by the power), I plug it into Mathematica/Sympy/whatever, or [most likely] I use a numeric integration algorithm.
There’s perhaps more value in memorizing the techniques to solve various forms of differential equation by hand, but even then your “real” implementation is ultimately likely going to be numerical.
Knowing integration formulas is really important when looking for a way to get the integrand into a form that works well with eyeballing the integral or numeric integration. It also lets you estimate early on how hard actually finding the integral will be (spotted some terms which don’t appear in any of the formulas? Then the problem might be of the tough or unsolvable kind, if the terms cannot be rewritten in terms that appear). The more you know, the better your estimate will be.
Sure, but there isn’t a ton of value in memorizing the sort of puzzle integrals of sines and cosines multiplied by polynomials etc you see in intro calculus, which I’ve seen people fixate on. It’s something you learn once and then file away in a mental bank so that you can recognize when you need to go look up the details of some trick in a textbook. There’s not a lot of deeper insight into memorizing tricks longer term, you miss the deeper insights of math.
There’s not really a “mental bank” you can rely on, because the details get lost over time. You use it or lose it.
I’ve lost the use of double integrals by now, and re-learning it would take so much time that when I need them, it’s faster to rely on other methods.
As a teacher (formally and informally) it’s always difficult to predict what learners will need in the future, but in general I try and go with the idea of ranking things in descending importance from “foundational” to “you should know how but usually look it up or let a computer do it” to “good to be aware of”. Aside from this there’s some fun stuff with probably no real applications for non-experts (I will never need to know how to lure a giant squid, but it’s kind of cool to know anyway and can be good context for foundational concepts like problem solving or food webs). Arithmetic up to 20 tends to be foundational, where long division tends to be something I think people should be able to explain at some point but usually hand off to computers and cube and higher roots are generally good to be aware of, but not something most people are going to need often, or even need to technically know how to do. Obviously YMMV for anyone regularly using calculus, but I think anything beyond adjusting powers with integrals and derivatives is going to be in the group of things often best left to computers or lookup once you go through the steps a few times to understand that it’s possible to do, or to be aware of as a possibility and then go look up in the rare instance it actually applies.
Part of calc 2 was just learning to use the table of integrals to solve more complicated integrals. No memorization, and much more about learning the patterns to solve integrals (integration by parts being the best known).
I integration by parts kicked my butt, initially. But at the end of the aemester, we were snowed in and couldn’t leave. I walked to McDonald’s and got a QPC. Then, I unboxed my Calculus book and proceeded to teach it to myself. It was one of the proudest mkments of my college career!
I found that if I memorized the unit circle, I could figure out sin/cos/tan. And if you know that sin(0) is 0 and increases as x increases, and that the max slope of a sin wave is 1; the slope of sin at 0 must be 1: derivative of sin is cos. So the integral of sin(x) must be cos(x). Plus a constant.
Being a mathematics teacher (both pure and applied for over 20 years), I feel like the author is playing fast and loose with some of their language. “Wrong”? Not quite. “Incomplete”? Yes. I’m sure that the tone helps with the YouTube algorithm. Sure, recognizing when and how to use integration in applications is an important skill that a lot of students struggle with. Teachers should help their students develop and hone that skill when teaching applied mathematics. But, integration is a far larger topic and is not merely a tool of arithmetic. There are many areas in mathematics where assuming that a purely concrete, arithmetic interpretation of the integral can become more of a hindrance. Not all mathematics is on objects with physical analogies.
Developing logical skills for problem analysis and solution synthesis is usually more productive for students that rote formalism. I will agree on that. Whether or not being able to solve some test questions is an indicator of how deep students are comprehending deeper mathematical concepts is another issue entirely.
It’s an interesting video that I intend to share with my colleagues.
I find that students who learn the “big picture” at the expense of rote formalism tend to get bogged in the details when it comes to actually performing the math, and shy away from actually using it even though they might know the gist of how to solve some problem. After a while, since they’re not using their skills for problem solving, they tend to forget the logic and analysis as well – and that’s when math becomes hard again.
A good example is students who whip out their symbolic solver calculators the moment you ask them to solve a second order polynomial – in college. Take away the calculator and they just stare at the paper with a blank expression until you give the answer.
When I was still undergraduate, I once forgot to bring my calculator in a chemistry exam. I just did the calculations long on the back of the paper. That would be an impossible ask with the students I see today. They are taught the algorithms for division, multiplication and addition, but they stop using them after grade six, so the routine goes away and they simply forget it. With modern calculators and math software, and online tests where you don’t need to write down your work, the same is happening on things like solving equations analytically. Students see the expression and if the calculator doesn’t solve it, they just plop the whole thing in and start trying different values of X.
That has been a persistent problem in my differential equations classes over the last few years. At first, it was just a few students with TI-89s. Then the phones started getting more capable. For the last few years of freshmen classes, it has been like that line in the movie Tron “the computers will start thinking and the people will stop”. About ten years ago we instituted a policy of requiring all work to be shown on exams. That helped a bit, but as you indicated, they can’t show steps that they don’t know to do.
I’ve been going back to Polya more recently and emphasizing the value of sketching and concretely explaining your way through to an understanding of a seemingly complex problem. I’ve also been handing out larger reference sheets of seemingly obvious identities. Both have had positive effects. An even more effective approach this last year has been showing more useful tactics for layout of hand-work on paper. Like general note taking, not everyone gets taught how to organize information on a page. Legibility of hand writing has declined too, but that’s another problem.
https://en.wikipedia.org/wiki/How_to_Solve_It
One problem we’re facing right now is that people forget to do unit analysis, since the automated homework checkers used in online platforms only require you to write down the numerical answer, so that’s been lost as well. Students get wrong answers and don’t know why, where if they were doing unit analysis they’d see that the answer comes out as square root of Amperes instead of Watts etc.
If you know the physical definition of the answer W = J/s, and you know that V=J/C and C=A*s then you can work backwards to say that W = VC/(C/A) = VA and check that against the formula you’re using – but doing so requires knowing how to manipulate such expressions. Many students already forgo the fact that 1/(1/X) = X and instead enter the more complicated expression in their calculators, which is where the errors creep in.
That’s an excellent point. I too struggled with the big picture in my 3rd year at university. It took a lot of grading papers as a TA to recognize the value of being able to just apply the patterns and complete the required calculations. These days, my colleagues and I try to balance curriculum between “math as an art class” (discovery, expression, and meaning) and “math as a shop class” (making stuff that works and not loosing fingers on the tools”. Keeping student’s motivated to revisit fundamental skills can be difficult, but it’s use it or loose it.
A video about the Riemann integral, essentially. Too bad it never mentions Riemann sum a single time in this video at least for the reference.
My thoughts as well. Back when I first took calculus, we first learned about the Riemann sum, then segued into integrals.
The calculus class I took started with Riemann integrals and would typically go back to them to show what sort of section were being added to calculate things like volume or surface area. I’m surprised other methods would start elsewhere.
This video is bad on a number of levels. But at its most basic, Calculus is taught from multiple angles by teachers (all three that the video lays out). Often, the motivating example for integration is exactly the video’s #3 way of describing integration. I.e. taking measurements of rate quantites and turning them into aggregate quantities by summing with smaller and smaller intervals (i.e. speed to distance with 1 sample a.k.a average speed to distance). In fact, a great way to describe calculus is the process of being able to map between rates and aggregate quantities. But my thesis is that you need all three of the interpretations in the video to really benefit from the study.
But why calculus is useful is not just repeated summing, it is the mechanical tools to do these analyses, i.e. integration and differentiation operators in an algebraic setting. This gives you the ability to go beyond a numerical setting. You can argue with a computer the analytic setting is less important, but usually it’s that analytical tools let you develop better algorithms. For example Riemann summation gives way to trapezoid/Simpsons rule but then there is a whole field of quadratures that are all derived from analytic math. So antiderivatives are to repeated summing of measurement like algebra is to using a calculator to compute expressions over and over again. It’s not useful to observe you are solving an integration problem unless you are going to use the numerical and analytical tools of integration.
Lastly, the area under the curve is another useful way of thinking about integration that allows you to see how integration works in the graphical setting. The graphical analogy is a way of developing intuition outside of algebra.
All and all, you were taught Integration wrong if you weren’t taught from all these angles. I think it is worthwhile to teach integration and differentiation from the application side. You can even argue most people don’t need to know about the manipulation and derivations of Calculus, but if you don’t learn those you aren’t really learning integration. You are learning the motivation of Calculus.
On the other hand, click bait titles do attract people to revisit ideas and concepts that if they had technical titles wouldn’t attract them. If they had titled the video “Caclulus educators should de prioritize the graphical and algebraic form of integration in favor of the Riemann integral and numerical quadrature” nobody would watch the stuff. I recently was having dinner with two top-tier educated engineers, and they made the argument that math was overrated and they never used it in their daily life.
If you are out to think about how to solve problems mathematically you will find applications of algebra, optimization, differential equations, calculus, and of course statistics. You will use it everyday, and your solutions to your live’s decisions will be easier. Effective users of math move between algebra, graphical, and specific example representations rapidly and effortlessly.
I agree completely. Like I tell my students, mathematics knowledge is always being expanded and revisited. There are hundreds of proofs for Pythagoras precisely because mathematical concepts can be approached from so many different, and often unexpected, ways. By studying and following different approaches to a particular mathematical concept, we deepen our understanding of that concept and find new applications for it.
Applied and pure mathematics are useless without each other. Period. As a practicing engineer and math teacher, I strongly believe that any engineer who shuns the value of mathematics in principle is a danger to society. Mathematicians who scoff at applications are also a hazard, but they tend to build fewer proverbial bridges to fall on people.
Hmm…. I’m going to wait till I’ve had a few cups of coffee to see what this is about. I’m pretty sure that summing progressively smaller bits is exactly how integration was introduced when I took it so… coming in skeptical.
Ok. finally watched it.
So… I know my calc teacher went over this.
Is that because it was 20 some years ago and.. new math?
Was it a particularly good calc teacher?
Or do most go over this and the video is a response to some outlier?
Calculus. You’re doing it wrong.
Headlines like this are why I don’t have social networks or any interest in this clickbait nonsense title. The comments show that I’m not incorrect on this. I had a longer comment but I’ll leave now.
I agree with the previous commenters that this clickbaitisms should not be tolerated. I usualy don’t watch such videos (if I am able to recognize that they are just clickbaity) but if I am lured to watch them I always put a dislike – this is the only circumstance I put a dislike – if I’m mislead by the title.
I respect the effort people put into this
Actualy I was taught integration with first Rieamann sums, then with Darboux sums (or it was the other way around), then I was shown that the two deffinitions are equivalent.
But this is not the point. What I wanted to say is that there is no wrong way of teaching. You either are able to transfer the knoledge or you don’t. And usualy the more ways of showing something – the better the understanding.
The second half of calculus 1 and first half of calculus 2 covers a lot of calculus applications, most of it is using calculus to solve a variety of problems. This is required for all 4 year science degrees where I live.
I liked this guy’s explanations:
https://betterexplained.com/guides/calculus/
Idk about being taught “incorrectly” but Simpsons Rule saved my bacon on the Professional Engineers exam! Having that programmed into my calculator saved me tons of time!
Everything old is new again. This wonderful book helped get me through Electrical Engineering many year ago.
https://www.gutenberg.org/ebooks/33283
grumble there’s no ‘there’ there. the #3 minute summing *is identical to* the area under the curve #1. that’s how it’s always taught. estimating the area first with summing and then limit as delta x goes to 0. most exciting lecture of all of highschool. why when i was young blah blah
What I lacked in math class, is background on the way techniques were developed and *why* they were developed. New material is just presented as-is, but in reality it did not appear out of thin air, but was the answer to a problem. Newton, Gauss and others weren’t just making things up. They tried to solve practical or academic problems.
If you understand the problem, the solution makes more sense.
Back in the mid-80s, in the UK, our ‘AO’ (midway between ‘O’ and ‘A’) and ‘A’ level SMP maths courses taught integration by starting with the trapezium rule for integrating polynomials; and seeing empirically what the results become, in order to derive more general rules of integration. Also, my calculator, a Casio Fx-180p could do definite integrals anyway via Simpson’s rule.
https://www.calculator.org/calculators/Casio_fx-180P.html
The 180p didn’t have hyperbolics, but the 38-step program area could implement them pretty well. And yes, we were allowed to use a programmable calculator for exams if we proved the program memory was erased (by removing the batteries) before the exam.