
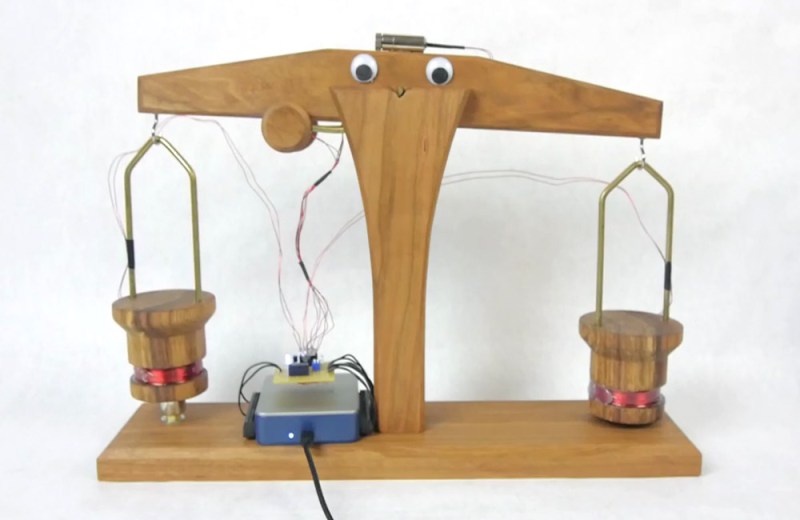
Machined from a chunk of a virtually indestructible platinum-iridium alloy, the international prototype kilogram (IPK) was built to last for an eternity. And yet, being the last remaining, physical artifact in a club of fundamental SI units, the current definition of the kilogram has worn out. Most certainly the watt-balance will take its place, and redefine the kilogram with a true, physical phenomenon. [Grady] just built his own watt-balance from scratch, and he provides you with a decent portion of scientific background on the matter.
A watt-balance doesn’t look or operate much different from a regular balance, only instead of weighing an unknown mass against a calibrated counterweight, a watt-balance employs a calibrated electromagnetic counterforce. That force is produced by an electromagnetic actuator. [Grady] scratch-built his watt-balance from wood, however, even though he carefully turned and wound his own coils for the electromagnets, he cannot be quite sure about the exact amount of force his actuators exercise per ampere of consumed current. Before a watt-balance weighs redefined kilograms, the contraption needs to be calibrated.
Thanks to algebra and physics, this can be done by measuring the voltage the electromagnetic actuator itself generates when its permanent magnetic core is moved at a given velocity by an external force. Therefore, the watt-balance features a second, electromagnetic actuator with optical position feedback (practically a servo), to slightly rock the balance back and forth. [Grady] realized the optical feedback by installing a shadow sensor, made from a line laser and a photodiode, to the arm of the balance. An Arduino Uno polls the sensors, samples the induced voltage and sends the measurement data to [Grady’s] host software. After being calibrated in this velocity mode, the balance is operated in its weighing mode, where the gravitational force of the mass in question and the electromagnetic force exercised by the actuator are brought to an equilibrium by simply adjusting the current through the electromagnet’s coil.
We’re glad to see these mighty instruments being built from wood or even LEGO. Enjoy the video below, where [Grady] walks you through the engineering behind his watt-balance.
















Wouldn’t the results be changed if the (metal) object on the scale is able to interact with the magnetic fields below it? Should the isolation be greater than it is?
But, but, the googly eyes could settle off center a millimeter or two either way.
Better than following you as you walk around the room….
And thus was reinvented the force restoration balance, the principle of which is behind every lab scale capable of resolving more than 10,000 counts over its capacity range (that being the cheaper province of strain gage load cells).
I don’t believe he’s claimed to invent anything. He just DIY’d his own balance and explained the technology.
Err Why not have the 2 E-magnets on the base and have the Metal hanging ?, Hiding the wire with less obstruction ?.
The ferrous material needs to be in a fixed position. Movement may alter its interaction with Earths magnetic field, or nearby external fields.
have you built a gravitational wave powered motor yet ?
Proper design and forethought are critical in creating a reference instrument.
“Most certainly the watt-balance will take its place…”
Well, there’s still the Avogadro Project, too.
These guys are counting silicon atoms instead.
Wouldn’t temperature affect the current, and therefore be a variable that needs to be accommodated for?
I suspect the best fit modeling helps with this. Thermally controlling the apparatus like in the case of OCXOs would be the next logical step to improve that. The trouble with full control over thermal properties I suspect is which part do you directly control? Direct accurate thermal control of the scale side would seem like the hard part of this to me. Invert the setup so the magnets are free and heat or cool the coils, or compensate the magnets to maintain their properties? How far could you get with superconductors?
The current needs to be well regulated.
The idea of a balance is that when you have 2 masses, it doesnt matter in which graviational field you are, the gravitational acceleration on both masses are always the same. This means that you dont only have to calibrate for the force of the actuator, but also for the gravitational pull on that moment.
In other words, this device estimates the pull on the mass and not the mass itself.
So the definition of mass would be restricted to one location on earth, since gravity is not constant at all points ?
Mass = how much stuff is in a thing, weight = the force gravity pulls that stuff with. So things have different weights under different gravity, but always the same mass.
Oh hang on, so yep, this thing measures weight, not mass. So you’d have to define a standard place to calibrate it from. Though does gravity vary all that much on Earth? I suspect the limit on this thing’s accuracy is not varying local gravity though.
I dunno if that was taken into account when designing Le Kilogram Internationale, again, probably less than they could measure. The new definition of so-many silicon atoms will be immune from that though, since that’s real mass.
In space, in zero G (actually freefall, but it’s equivalent to zero G, even though it’s pretty much 1G), they measured the mass of Skylab astronauts by rocking them back and forth in a chair. The inertia from their mass was measured and used to “weigh” them for checking their health.
It’s a balance, not a scale, It measures mass.
Surely it measures the downward force of an object, caused by gravity. It wouldn’t work in 0g, and would give 1/6 results on the Moon.
It’s a force comparison. It uses the force of the electromagnet to resist the force applied to the mass by gravitational acceleration. It measures current as a substitute for measuring force. Put this in an accelerated frame of reference and it will misreport the apparent mass because of the changed force.
Gravity is generally consistent to at least one decimal place but if you’re on a high mountain or over a dense ore body you may weigh a bit different than you do on your bathroom scale.
Gravimetric scans are useful in quite a few industries from mining, hydrologic surveys, to defense purposes since large voids holding soldiers & missiles weigh different than equivalent volumes of bedrock.
There sure are a lot of folks these days who don’t regard actually watching the video relevant to commenting on it. The guy explains rather clearly that yes, the local value of “g” needs to be known for it all to work, but we do have unrelated methods to measure it very precisely.
I find it hilarious that SI units are every bit as arbitrary as imperial units, and that in each case the people in charge had to find some way of defining them as something other than themselves. So really the only difference is that one system is base 10 and the other isn’t. Big deal.
From now on, I think people should only use the following units:
Furlongs per Fortnight – velocity
Attoparsec – Distance
Smoot – Length
Pirate Ninja – Power consumption
Shakes of a Lamb’s Tail – Time
And so forth.
Imperial units are just SI units with window dressing; for example the inch is defined as being exactly 2.54000 centimeters. You’re welcome to work in base 2.54 if you want to but 10 is a bit easier to deal with.
You’re aware that has only been the case since 1975 right? Metric has been in use for surveying since the 1820’s and formalized in the 1860’s but it was not the definition of any imperial units.
With similar changes to UK law in 1978 & 95.
As for using a metric base given the current definition, I fail to see your logic, it doesn’t even make sense mathematically. Sure 1 inch = 25.40 mm but fractional or even (gasp) decimal inches provide all the detail needed for even precision tasks.
For the most part they are not: 1W is exactly 1J/s, 1J is exactly 1Nm, 1Ohm is exactly 1V/A etc. . Well, those are the derived units, the base units are indeed ‘arbitrary’ in a sense (much thought went into which should be base and which should be derived units). What do you propose instead? You got to draw the line in the sand somewhere.
As others commented on already, base 10 is a big deal for humans. Further some of the choices made are practical: a volume of (0.1m)^3 holds 1l, which, if filled with water under normal conditions, happens to be pretty close to 1kg, etc. .
And, uh, the rest of the world is using the Si system (in trade at least) …
So, a bunch of arbitrary units, loosely connection to those from history, that have no mathematical relation to each other? That sounds a lot like Imperial already.
Actually the Unit size for C is less useful than F. The only problem with F is that they zero point is wrong.
Having 180 degrees between freezing and boiling is a lot more sensible than have 100 degrees.
Nah, we should just set the Planck temperature as 1 and absolute zero as zero.
Just plain stupid. A scale needs to be a multiple power of its base. Typical stupid colonials…
Why is 180 better than 100? It’s harder to do maths on. You mean simple because it’s bigger? We have decimal points for that, and everybody, even norms, understand that. Or do you plot a lot of temperatures as circles?
180 isn’t better per se, but 360 is a highly composite number. It’s practical that it’s divisible by 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 45, 60, 72, 90, 120, 180, 360.
But I wanted to divide by 7
Pah, pathetic weaklings! Nothing less than a proper, Pi-based unit system will do! You yanks are welcome to go with the square root of two if you really must…