There are many well-known physical constants, but it always interests us when someone can approximately measure them using equipment you probably have. We could pretend it is because we want to help kids do science projects, but who are we really kidding? It is just the cool factor. [Stoppi] shows us several neat ways to measure Planck’s constant (German language, Google Translate link) using things like LEDs, solar cells, and common test equipment. If you don’t want to translate the web page, you can also see the setup and the math behind it in the video below.
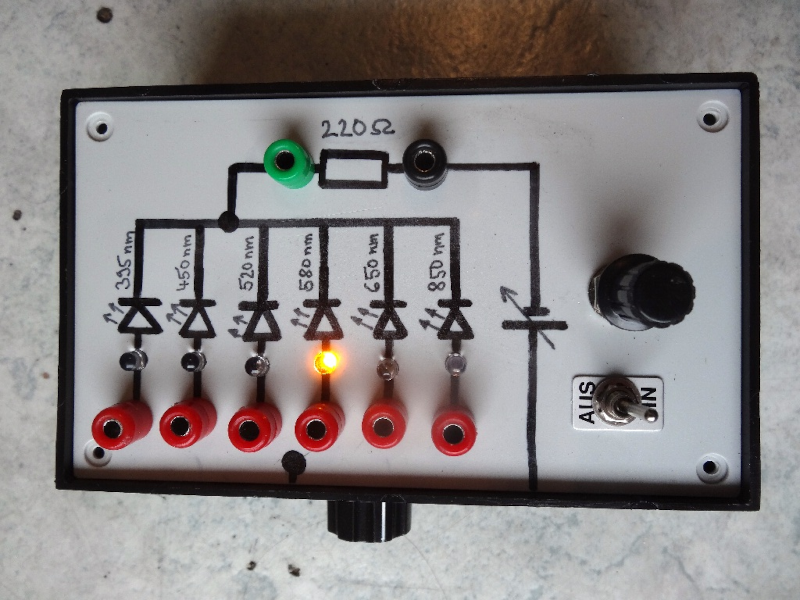
If complex math triggers you, this might not be the video for you. The particular test in the video does require a very low current measurement, but that’s not very hard to arrange these days. There are actually several methods covered in the post, and one of them uses one of those familiar “component testers” that has an Atmel CPU, a socket, and an LCD. These can measure the forward current of LEDs, and if you know the wavelength of the LED, you can determine the constant. There’s even a custom device that integrates several LEDs to do the job.
The method in the video is actually the fourth method in the post, using a light bulb and a solar cell. That method uses an optical bandpass filter. The experiment used two different ones and the 785 nm filter provided a good estimate (5.62×10-34 vs the actual accepted value of 6.626×10-34). While 5.6 vs 6.6 might not seem that close, note that this is an extremely small number, so being off 1×10-34 isn’t very much.
The custom box method was even closer. The single LED and component tester methods were fairly close but off by a bit more. The custom box reminded us of another custom instrument for this purpose, the Star Trekesque named “photon energy array.” The other method we’ve seen used Lego.
















What if I don’t ‘need’ to translate the page ?
Then maybe you could chose which language you feel like reading it in. Is there anything else I can help you with today?
Then you click the non-translated link, pretty obvious, no?
“While 5.6 vs 6.6 might not seem that close, note that this is an extremely small number, so being off 1×10-34 isn’t very much.”
The absolute value is irrelevant, since it depends on arbitrary definitions of the SI units. What matters is that the calculated value is ~17% too low.
Nonetheless, an interesting article, particularly because it compares several methods and provides the physics :)
Diodes are not a good way to determine the Planck’s constant because of the interface effects between the p and n regions. So applied voltage times e will not necessarily be Planck constant times frequency.
https://iopscience.iop.org/article/10.1088/1361-6552/ab525d
You are correct on your assessment of the irrelevance of the absolute value. And considering the simplicity of the equipment and approach, 17% error might be considered pretty much spot-on.
Back in my teaching days the gen-chem students did several experiments—determination of the gas law constant, absolute zero, Avogadro’s number, etc.—where 17% error would be awarded full credit. For the (admittedly crude) Avogadro-number determination, an answer that was within an order of magnitude of 6e+23 was quite acceptable. Off by *two* orders of magnitude? 5% penalty. :)
“being off 1×10-34 isn’t very much.” Actually depending on which way you go it’s 16 – 20%. Grading a student is one thing, for the writer of the article of the article it’s an egregious error and suggests sucking.
This is grade school math dude.
[english] Ahh the good old Aldi Battery.
[Deutsch] Ja mei, das ist ja die gute alte Aldi 9 Volt Zelle. :-)