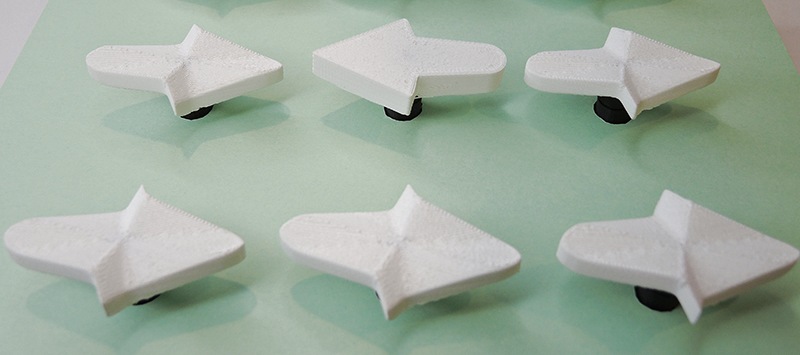
Last year, mathematician and professional optical illusionist [Kokichi Sugihara] came up with an arrow that only points one way. Technically, it’s ‘anomalous mirror symmetry’, but if you print this arrow and look at it juuuussst right, it appears this arrow only points one way.
[Ali] had the idea to turn this arrow illusion into something motorized, and for that he turned to 3D printing. The models for the illusion arrows were already available, but there had to be a way to turn a single arrow into an art installation. For that, you just need a few 9g servos. [Ali] slightly modified his servos so they would turn a full 180 degrees, and designed a magnetic mount to allow easy swap-out of these arrows.
The servos are attached to a 3D printed frame with heat-staked threaded inserts, and driven by a Pololu servo driver. The effect is great, with multiple arrows twisting and turning but still only appearing to point to the right. [Ali] put together two videos of this arrow illusion, one that’s effectively a build guide, and of course all the STLs are available in a link in the description.















Just what we need when dropped means added. Digital state becomes mud.
i just dont get whats the point of sticking this illusion on an array of servos? (Dont get me wrong, the illusion itself is awesome but what does the number of servos (and the arduino) add to it?
I know, they could have done the same thing with some 555 timers.
I agree, to me it makes the illusion less impressive to have so many of the arrows flipping around and dancing.
I like Sugihara’s earlier illusion better: https://www.youtube.com/watch?v=oWfFco7K9v8
I found the paper for this here: https://pdfs.semanticscholar.org/e620/9b9221425fdd553091c1c19bf9bb9cd5ed6d.pdf
That’s actually about yet another illusion, which makes shapes appear to intersect from one direction, while being separate from another direction. Not quite as cool from where I look, because the slightest move from the ideal position breaks the illusion. This looks like something done on a dare. You know, “Okay, changing the shape of something is one thing, but can you separate linked rings? Hmmm?”
You can notice the same thing with 2-bladed aircraft propellers viewed from an angle near to the pitch angle of the blades.