A few weeks ago, we looked at a video showing water “solving” a maze. [AlphaPhoenix] saw the same video, and it made him think about electrons “finding the path of least resistance.” So can you solve a maze with foil, a laser cutter, a power supply, and some pepper? Apparently, as you can see in the video below.
At first, he duplicated the water maze, but without the effect of gravity. It was hard to see the water flow, so pepper flakes made the motion of the liquid quite obvious. The real fun, though, started when he cut the maze out of foil and started running electrons across it.
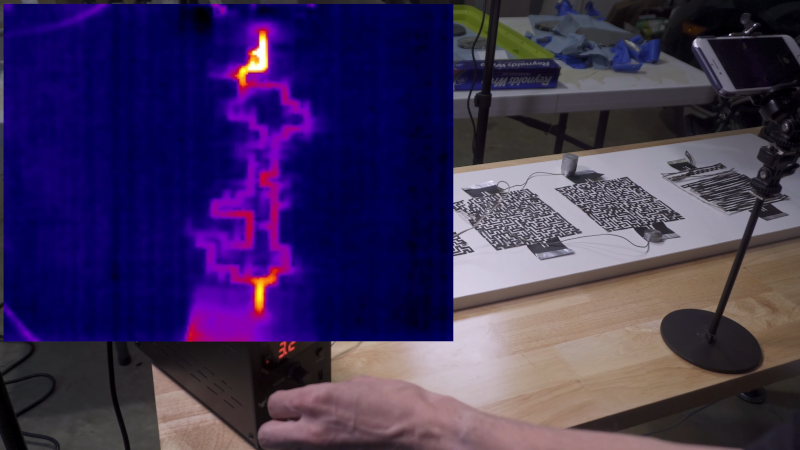
It isn’t easy to visualize electrons, but you can see the heat they produce using a thermal camera. Of course, a physics guru will tell you that you really aren’t watching electrons flow, but rather you are seeing charge moving via charge carriers. Regardless, the effect is that electricity flows, and you can see how that works with the thermal camera and develop intuition about it using the water model. A cool demo.
If you want to watch the video that inspired this one, we covered it. If you didn’t get a thermal camera for a gift last year, you can buy one for yourself, but be sure to check out the comments for some options the post didn’t cover.















I don’t get this.. As far as I can see, there’s no “maze” there, there’s only one connection between the two ends, it’s just one large odd-shaped conductor?
This and the water experiment are not really “solving” the maze. That’s just a conceptual slight of hand.
Consider, if we were to paint the right path with a fluorescent dye and then shine it with UV light, does turning on the light “solve” the maze, or simply reveal the answer?
Shining a UV light from above isn’t what’s going on here, though. In that case the light is just revealing the dye, it’s not following a path in the maze.
Looking at the DC result at the end is the wrong way to think about it. If you imagine using a fast enough time-domain reflectometer on the maze, you would see every single fork and dead end in the maze. Of course, in this case, it’d be jumping over the “walls” too, because electromagnetism doesn’t care about your silly rules, but in the conceptual limit (buried in an extremely high dielectric material) you’d see reflections off of every wrong path.
That’s why he pulled out a scope in the video, but of course there’s no way in that setup that you’d get any result – at GHz frequencies, the electric field just says “yeah, imma gonna just take this air path, it ain’t that bad.”
In the electric current case, the current is just revealing the EM field gradient that already exists before the current picks up.
>Shining a UV light from above isn’t what’s going on here
If you wish, you can imagine yourself going over the maze with a tiny spotlight UV lamp – makes no difference. You’re not solving the maze, the maze already gives you the right solution and you’re just following along.
That’s all a maze is. Just one large odd-shaped path from in to out.
Without the possibility to take a wrong turn and get stuck or lost, there is no maze. Take a straight road and make sharp bends in it – does that make it into a maze?
A road with bends and dead end branches is a maze. To the eye, the metal paths in the video are a maze. Current flow “knows” which branches are dead ends, and only flows through the one real path through the maze.
The current flow doesn’t “know” anything. It’s just not possible for it to take paths that have no voltage gradient. When you connect the sources, a voltage gradient emerges along the correct path, and the current sees only this path – the rest of the “maze” doesn’t exist for it.
Compare and contrast to the situation where we have painted the correct path with UV markers and look at the maze in a dark room with a UV lamp. We can only see the correct path and none of the branches.
Mind, you have to look at it from the perspective of what is solving the “maze”. A maze (as opposed to a labyrinth) has the structure of a tree with right and wrong branches you can choose. For the electric current, there are no branches: it takes all possible paths simultaneously, so there are no choices and the solution is already built in.
It doesn’t, actually. It just does it so fast you think it’s simultaneous.
When the circuit’s first connected, the field that rushes through the circuit *does* branch off. Those open bits are stubs off a line, and they *do* get field in there until they charge to the same potential and stop. You get reflections off an open stub, so you can’t say it “doesn’t take it.”
At DC, the structure of a wire doesn’t matter, but at AC it absolutely does.
> It just does it so fast you think it’s simultaneous.
In terms of the EM field, it already exists even before you connect the wires. The EM field “feels” its surroundings all the time and it already “knows” where the current should flow. Actually, some current is already flowing, but very little because the conductance of air is so weak. The “maze” is already solved before the current picks up, and the transient currents that follow when the wires are connected have nothing to do with it.
“In terms of the EM field, it already exists even before you connect the wires.”
You’re thinking of the DC case. If you put a voltage pulse in, there’s no “pre-existing” electric field. You start the field, and it spreads through the maze, branching at every option.
Btw. an un-ambiguous convoluted path that folds on itself is called a labyrinth, though the words maze and labyrinth are often used to mean the same thing.
For me, for it to be a maze, there must be a wrong way, it must be physically possible to take a wrong turn. There’s no electrical maze, electrons have no option to take a wrong turn, there’s nothing to do in this conductor.. The electron migration path actually show this.. there is a tension between the two connection, so the electrons near these has to move, thus moving all electrons between the contact points, this creates a flow along the path. All the other electrons do absolutely nothing, they don’t move or take any paths, there’s no tension.
That’s not true. They *do* take the wrong way, they just do it extremely, extremely fast.
Think about the simplest maze (not even a maze) – just a straight section, entrance to exit, a simple wire. Put a potential across it, the field sets up basically straight.
Now put two dead-end branches off that (so like, a cross, with entrance at top and exit at bottom), and do the same thing. Do you get the same result? *No*. The current *splits evenly* at that junction, just like water in a vacuum with no back air-pressure. One third of the current hits the exit right away. The other two thirds take the dead-end paths, and “fill up” those paths, so *new* current can’t take that path – which means for the next chunk of current, the full current gets through.
So how can you see this? Think about what happens *in time*. The difference between the straight section and the branched section is that in the branched section, it takes longer for the full stream of current to hit the exit.
And that’s because the branched wires have more capacitance. Which slows down the risetime of the signal.
The electric field gradient that solves the correct path is already established before the wires are connected, because a voltage difference exists between the two wires and all the charges in between experience their influence accordingly. Connecting or disconnecting the wire simply determines how much current can flow, so a sudden change causes a transient to “slosh” around for a moment.
That doesn’t mean the electric current “takes the wrong path”, as if it were seeking the way through. In terms of the water analog: you already and always have SOME water flowing through the maze by the very setup of your system, so the correct path is already established, and any more water just has to follow the same slope.
In fact, the water analog that the guy uses in the video is highly misleading, because the maze starts off empty. The metal conductor is not empty of electrons – it’s full of them.
A better analog would be to level the water maze and fill it up half-way with water. Then, as soon as you start adding water at the entry, you also lower the gate that kept the water from spilling out the exit. Then you can see, while for the top half of the gradient, the added water starts filling the dead ends, for the lower half the water actually flows out of them, and for the middle part nothing changes and the water simply flows the right way – so is it actually the water that solves the maze, or the maze that solves itself?
“The metal conductor is not empty of electrons – it’s full of them.”
Yes, this is the misleading part. It’s the electric field which is racing through the maze. The electrons barely move. Everyone makes this mistake.
I don’t understand why you keep saying that it’s “already there.” If there’s no voltage, there’s no field, there’s no current. Don’t think of “connecting the wires.” Start off with them connected. No voltage. No field. You put a pulse in, the field will race through the maze, reflecting back (with same polarity!) off of dead ends, and causing ripples in the field.
You obviously can’t do this with a strip of conductor in air, because the field just looks at the air and says “yeah, that ain’t that hard” and jumps it. But if you took, say, a 4 layer PCB and made a maze on two inner layers with opposite polarity (so you’re driving it differentially), had a high dielectric material such that the common-mode impedance was much higher than the differential, you could *easily* see the signal bouncing off of dead-end paths.
This is just time-domain reflectometry.
It’s a “maze” because there are dead ends, and other options for something other than electricity to fall into.
The electricity is a tool that “solves” this maze quickly using it’s unique properties.
If you were to create a “maze” with electrically conductive channels that you do not know the answer to, the electricity can show you the answer quickly.
Just because the tool you’re using can solve it quickly (near instantaneously in this case), doesn’t mean that the maze doesn’t exist outside of that tool’s properties.
Its cool to see how they inspire eachother, awesome concept :D
This is a heuristic computer that solves a problem by first finding the wrong solutions. Using electrons instead of water makes it find the correct path almost intantly.
What I eny most is having the free time to do this !!!.
It’s not heuristic, it’s deterministic.
The claim is that electricity can solve a maze, not that it can solve the electrical equivalent of a maze. The mazes shown in the video are undeniably mazes. Give them to your kids and watch them go.
Maybe this is the intelligence behind ChatGPT?
“Water Solves Mazes, Why Not Electrons?”
Because they cheat! Just ask any Esaki diode!
Well, if I understand correctly we ARE seeing electrons travel through the maze. We’re seeing a raise in temperature caused by resistance in the conductor. The resistance is caused by flowing electrons colliding with atoms and transferring energy.