Logarithms are a common idea today, even though we don’t use them as often as we used to. After all, one of the major uses of logarithms is to simplify computations, and computers do that just fine (although they might use logs internally). But 400 years ago, doing math was painful. Enter Joost Bürgi. According to [Welch Labs], his book of mathematical tables should have changed math forever. But it didn’t.
If you know how a slide rule works, you’ll find you already know much of what the video shows. The clockmaker was one of the people who worked out how logs could simplify many difficult equations. He created a table of 23,030 “red and black” numbers to nine digits. Essentially, this was a table of logarithms to a very unusual base: 1.0001.
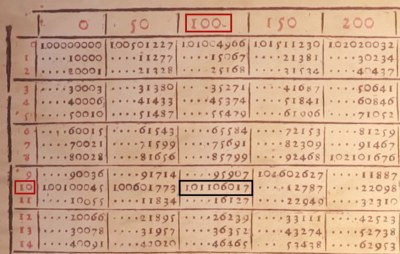Why such a strange base? Because it allowed interpolation to a higher accuracy than using a larger base. Red numbers are, of course, the logarithms, and the black numbers are antilogs. The real tables are a bit hard to read because he omitted digits that didn’t change and scaled parts of it by ten (which was changed in the video below to simplify things). It doesn’t help, either, that decimal points hadn’t been invented yet.
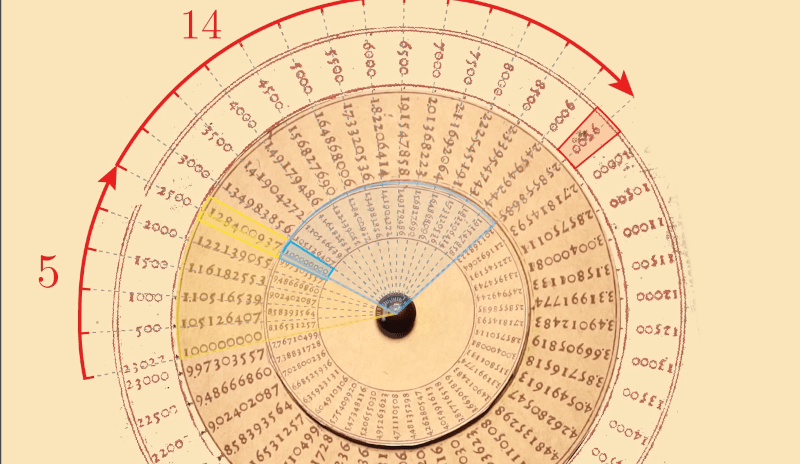
What was really impressive, though, was the disk-like construct on the cover of the book. Although it wasn’t mentioned in the text, it is clear this was meant to allow you to build a circular slide rule, which [Welch Labs] does and demonstrates in the video.
Unfortunately, the book was not widely known and Napier gets the credit for inventing and popularizing logarithms. Napier published in 1614 while Joost published in 1620. However, both men likely had their tables in some form much earlier. However, Kepler knew of the Bürgi tables as early as 1610 and was dismayed that they were not published.
While we enjoy all kinds of retrocomputers, the slide rule may be the original. Want to make your own circular version? You don’t need to find a copy of this book.
I don’t mind people going unrecognised, if they insisted if keeping works unpublished before someone more sensible comes along.
But what if that more sensible person doesn’t come along for decades or even centuries?
If he had the ideea of arranging the black numbers by theirs red equivalents on that disk he would get the first (circular) slide rule.
I don’t understand why log is better. Pencil and paper multiplication is absolutely trivial for even a grade schooler. Not particularly harder than addition. So why is adding logs so much “easier” than just multiplying numbers? Basically for ham radio that’s the argument for dB and… hard to imagine someone that can add easily but is eternally stumped by multiplication.
Especially if using logs means sorting through tables- itself very prone to error as I learned to use log tables and other archaic tables for celestial navigation.
Try square (or cube…) roots by hand.
Logs are useful because many practical problems don’t require a great deal of precision and also because it really is less tedious to add numbers than to multiply them. Further, you can raise things to a power or take roots of things simply by multiplying or dividing a log.
There are many things in real life, particularly fluid dynamics, that require fractional powers in equations. A classic example of this is the pressure differential relationship to flow in a Venturi flow meter. Further, taking the log of something on a graph can show exponential relationships as a straight line, thus making future estimations for things such as interest rates much more obvious.
Finally the decibel is useful because the numbers involved are often unwieldy. Perhaps the numbers themselves aren’t too difficult to multiply, but you can easily get the decimal misaligned. Many things such as a ratio are much easier to represent as a decibel gain or loss. And when you have many of them (particularly in Frequency Division Multiplexing) you get not just dB but things like dBrnC0 (decibels relative to noise with C-message weighting from the 0 dB Test Level Point). Also, the consequence of many calculations such as SWR are difficult to understand, but if you convert SWR in to return loss in dB, it makes a lot of sense.
I could go on. Yes, in today’s world, using a calculator is quite easy. But there are times and places where logs are still extremely useful.
Eh, Shannon-Hartley and a more convenient range of numbers if nothing else, as far as radio goes. Or anything else where a logarithm or exponent is already involved.
Adding *and subtracting* N-digit numbers one at a time from a running total is very routine; there’s a running total and a current operand. Dividing 123.45 by 9.8765 by long division is best left on paper instead of in your head, for proper precision. Multiplying is a bit easier, but still takes enough memory to really want paper. I would prefer a slide rule, especially a circular one, over a log table though.
Maritime navigation by paper chart still uses analog logarithmic computer/calculator “wheels” for speed-time-distance calculations. Old schools navigation guys will draw out a logarithmic triangle in a relatively blank space on the chart based on the charts scale for rapid math with a straight edge.
The circular grids used for plotting intercepts, called maneuvering boards or “mo-boards” for short, have common logarithmic scales used in navigation printed along their edges. The U.S. Navy manual on what all you can do with a mo-board is about three inches thick and lets you do rapid navigation and targeting math on the same sheet with wind and sea state factored in.
In training we would plot navigation courses around San Francisco Bay at 30 knots with planned intercepts of moving targets. We could “swing a fix” on a chart with three radar vectors and two visual reference points, plot two dead reckoning points, and advise best course to bring our gun to bear on the target in 60 seconds or less. Usually one guy on the chart, one on a mo-board, and one checking math. Could not have done any of that on the fly without the logarithmic scales and calculator-wheel.
Nautical slide rule: http://www.weems-plath.com/Calculators-Plotting-Aids/Nautical-Slide-Rule.html
Or in private aircraft, the E6B.