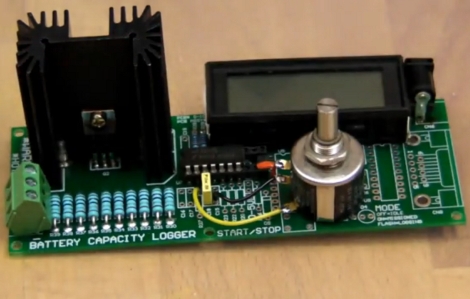
In [Dave’s] latest episode of the EEVblog he takes a look at constant current dummy loads. These are used to test power supply designs and instead of just chaining resistive loads together every time he’s decided to look into building a tool for the job. What he ends up with is a reliable constant current load that can be dialed anywhere from 1.5 mA up to just over 1A. There’s even an onboard meter so you don’t have to probe the setting before use.
It may look like he sent his design off to the board house for production but that’s actually a re-purposed PCB. In walking though his junk-box assembled dummy load [Dave] shares some great tips, like using multiple 1% resistors instead of shelling our for one large and accurate power resistor. But our favorite part comes at about 12:00 when he takes us through some rough math in calculating heat sinks. We’ve always just guessed, but like any good teacher, [Dave] explains the theory and then measures the actual performance taking the guesswork out of the design. See for yourself after the break.[youtube=http://www.youtube.com/watch?v=8xX2SVcItOA]
[Thanks Strider_mt2k]















Impressive, I learned something from one of his videos (aside from how to blow up a multimeter)
Finally, some one explains how the heat sync calculations work.
One thing is really misleading about the simplified calculations he’s using. For a passive (no forced air flow) heatsink, the *C/W rating applies only at one specific temperature. Normally it’s 60 degrees, but sometimes it’s defined much higher.
Why do you care?
Generally, the performance will be worse than calculated at lower power dissipation. Normally this is only a problem if you’re operating in high ambient temperatures. On the other end, the performance is better (to a point) for higher and higher heatsink temperatures. Be careful though, because there’s a point where your heatsink performance will suddenly plummet again.
Lesson: a heatsink manufacturer will give you a nice chart of temp rise / power. You should use this if your simple *C/W calculation puts the design close to your temperature limits.
Err… defined at one specific temperature rise*
Sorry.
lol, I watched his video on youtube a few days ago. was pretty cool actually. learned a lot.
Thanks for sharing. Was thinking of replacing my 2x12v power supplies(cable modem & router) for a single psu and maybe make something more efficient.
@Charper
I mentioned the calculations were back-of-the-envelope stuff, designed to get you in the ballpark. Yes, if you are doing serious thermal design then you have to go into the details, and they can get a lot messier than simply taking into account the thermal performance graph for the heatsink too!
Dave.
This is a neat hack, but you can do something similar but more sophisticated by using a FET as your resistive load: run it in its active range, rather than saturated. The FET is a *lot* cheaper than equivalent power resistors, it’s fast, and it dumps heat nicely. Plus it’s infinitely adjustable, as fast as you’d like it to go. Basically you use it as a current-sense resistor, and drive the gate with an op-amp that’s measuring the voltage drop across the FET. It’s a reasonably common technique and works beautifully. We use them as loads for testing LED drivers.
Point of fact, 10 10ohm 1% resistors in sereies does NOT equal one 100ohm 1% resistor, it equals 1 100ohm 10% resistor.
While probably still being cheaper than a production resistor it doesn’t give you the same confidence level, and thus cannot be used interchangeably.
@Leithoa
While you are correct when the resistors are connected in series, you have missed the point. The resistors are connected in parallel in this project. connecting 10, 10ohm, 1% resistors in parallel is, in fact, equivalent to 1, 1ohm 1% resistor which can handle a power equal to the sum of each resistors power rating.
phishinphree is right, in parallel it’s still 1%.
This is a very useful tool to have around and I think I’ll be building one. That said, the guy has a really squeaky voice that was almost painful to listen to.
A quick-and-dirty version: simply a powerful enough BJT with a heatsink, the base current (from an external power supply) is adjusted with a pot. An additional smaller BJT can bring the pot current significantly down. Occasional power supply testing seldom requires more.
I would love to scale this up for testing power supplies into the 10’s and 100’s of amps.
Would it be as simple as scaling up the components?
“Point of fact, 10 10ohm 1% resistors in sereies does NOT equal one 100ohm 1% resistor, it equals 1 100ohm 10% resistor.”
How do you work this out? I assumed that a 10ohm 1% resistor would be in the range 9.9 ohm to 10.1 ohm. Summing up 10 of those in series would give 99 ohm to 101 ohm, which would be equivalent to 100 ohm 1%.
Furthermore, unless the errors are all in the same direction, some of the errors would cancel out. I’m not sure what the correlation in error you’d get on a batch of resistors is, but I’d hope the final error would be less than 1%!
@Leithoa, @phishinphree, and others,
Is the tolerance of 10×1% series resistors really 10%? I don’t know the calculation, but it seems like those old stats concepts with standard deviations and bell curves would be necessary to find the total tolerance. It’s been >20 years since I barely passed that class and have only occasionally had to revisit that stuff since then.
Anyone know how to calculate tolerance stackup of resistors?
@sgf
You’re partially correct, you may get cancelation of errors. However there is now way that you can be CERTAIN that there will be cancelation. The point of the xx% rating is the manufacturer is guaranteeing that the product is at worst 1% off of the listed value, it could very well be exactly the listed value.
The formula to find the total resistance of a set of SERIES resistors is R=r1+r2+…rn and each of those resistance values is known to an accuracy of 1%. When a value with an uncertainty is added or subtracted the total uncertainty is found by adding all of the uncertainties together ie; {u}R={u}r1+{u}r2+…{u}rn. So for 10 1% resistors the uncertainty in the resistor value is R=1%+1%+1%+1%+1%+1%+1%+1%+1%+1%=10%
As was rightly pointed out by phishinphree and others the resistors are used in parallel, which uses a different formula to find the total resistance,R=(1/r1+1/r2+…1/rn)^-1. So given that we have 10 10ohm resistors in parallel we have R=10(1/10)^-1 =1ohm
So to find the uncertainty in that total resistance we must use a different formula.
{u}R=R^2 SQRT({u}r1/r1^2)^2+({u}r2/r2^2)^2+..+({u}rn/rn^2)^2)
{u}R=1^2 SQRT(.01/10^2)^2+(.01/10^2)^2+..+(.01/10^2)^2)=.000346 or +/-.03% for our total of 1ohm resistance. Which gives us GREATER tolerances than what we started with
These websites may answer more completely/clearly
http://www.ee.unb.ca/tervo/ee2791/errors3.htm
http://www.lhup.edu/~dsimanek/scenario/labman4/errorsim.htm
http://www.unf.edu/coas/chemphys/phys/physics2/lab/manual/Lab%204%20-%20Series%20and%20Parallel%20DC%20Circuits%20-%20Spring%202010.pdf
http://www.engr.uky.edu/~jdjacob/me310/Labs/exp3manual.pdf
@ Leithoa
I’m well aware of the properties of summing probability distributions – that’s why I mentioned it.
Thank you for providing links, but I’m afraid they don’t back up what you’re saying about summing relative errors.
I suggest you read up on the difference between absolute and relative error.
Indeed a rookie mistake, in my haste to recall the formula for the uncertainty in parallel resistors I skimmed over the fractional uncertainty tid bit, Good thing someone(SGF) was paying attention.
While trivial to fix in terms of arithmatic(Rs=.316%,Rp=.00316%), the real world consequences could be dire, kind of like when NASA forgot to use the same units for their one mars rover.
Excellent vid and info, thanks Dave.
…and your “squeaky” voice is champion. It’s so much more interesting and energetic than the dull monotone professor drawl that most of us EE’s are used to. good job.
For small runs, drift is far more important than tolerance. Constant errors can be trimmed out, or zeroed in software. For differential amplifiers, I start with 1% metal film resistors and bin them with a multimeter.
Ultimate guide to heatsink calculations:
http://www.mcmanis.com/chuck/robotics/projects/esc2/FET-power.html
applies to BJTs and resistors as well, just need to calculate power output a bit differently
bookmark it already