Summer in the Northern hemisphere means outdoor cooking. Matches are old school, and you are more likely to use a piezoelectric lighter to start your grill. [Steve Mould] has one, but he didn’t understand the physics behind why it works, so he decided to do the research and share it in a video.
The first two minutes is a recap of things you already know. But after that [Steve] gets into the crystal lattice structure of quartz. Using some computer animations and some peanut butter lids he shows you exactly why compressing the crystal generates electricity.
If you think you don’t care about barbecue lighters, [Steve] reminds you that the same effect is what makes the quartz crystals we use in crystal oscillators work. In particular, he looks at how a little crystal that vibrates at 32,768 Hz can make a watch.
We really liked the steel rule demonstration in the second video. It would be a great thing to do for a science class. The fact that the crystals replaced actual tuning forks inside watches shows how far things have changed in the last 50 years.
If you think you know about flip flops, by the way, you may find the end of the second a bit surprising, and quite the unusual use of flip flops.
We’ve looked inside old watches before. We also talk about practical applications of quartz crystals pretty regularly. But this is a nice set of demos and fun to watch.

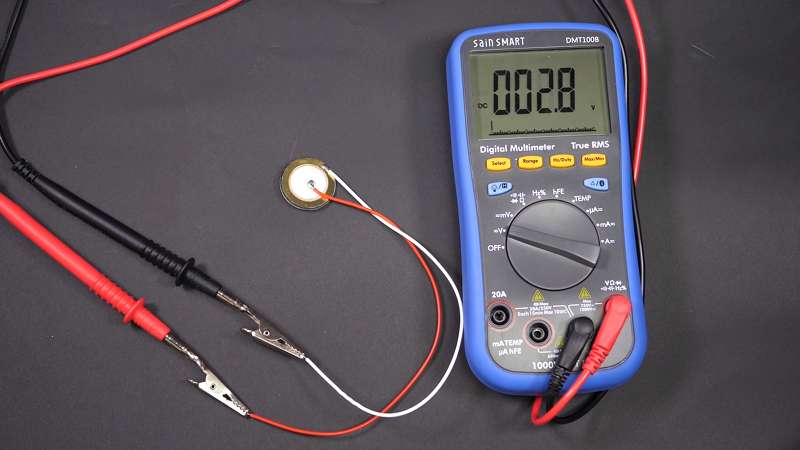














Peanut butter lids? They sell PB like pot? Technical words breakdown. Do you mean jar lids?
Only one watch ever used a tuning fork that I know of, Accutron. It was quickly passed by for quartz.
Well, I think most people understand the colloquial. As for the tuning forks, don’t feel bad. Most people only remember the Omega 300Hz. But in fact, in addition to Bulova who had at least the Accutron and the SpaceView) Tissot, Longines, PRIM, and a few other brands that escape me right (including at least one maker in Japan) used tuning forks. Bulova did invent it, though.
Remote controls.
Also Radio Control used an audio tone vibrating reed system for channel decoding from 50s through 60s. It was a component of “follow the beam” type radio navigation from the 30s.
As it says in the video, the quartz crystal is also shaped in the form of a tuning fork.
Not all quartz oscillators are like that, but if you want a “low” frequency like 32 kHz, you have to use the tuning fork shape because a simple tiny cube or sliver of quartz will have a natural frequency in the MHz range due to the high speed of sound in the material and the small dimensions. The relatively long tines of the fork provide an inertial damper that puts the resonant frequency way down.
AHSL Australian Hardware Synthesis Language
Five seconds a day for mechanical watches? Mechanical watches used on railroads were required to be accurate to within three seconds per week.
How were they tested for accuracy?
Excellent question! A (very) oversimplified answer is that you conflate “accuracy” with precision of the best instruments you have to measure the standard (if it has a physical standard). So if we were talking atomic clocks, you get a bunch of atomic clocks together and perform a statistical analysis on them to calculate the “average deviation”- this gives you the precision of your measuring instrument. The mean becomes your “standard” that all other accuracy is measured against.
Now, for the early days of railroad, I imagine major railroads actually had a central office that established this standard. Your average station master or engineer would then compare off that standard.
The topic of instrumentation precision/accuracy is covered in most engineering curriculua through your stats class (though since it’s usually taught by a statistics professor instead of an engineering professor, I doubt most engineering students even realize it). Good references in case you’re interested in further reading:
-Electronic Instruments and Measurements
-Errors of Measurement, Precision, Accuracy and the Statistical Comparison of Measuring Instruments – Frank Grubbs (highly recommended article)
Precision is the ability to tell the same time after a week or so. Accuracy is telling the exact time (e.g. noon) after a week or so.
You can compare clocks and establish an average to get precision, but not accuracy, because all your clocks may run fast or slow. That error would mean each clock would have to be calibrated to the same clock, and you’d have the problem of the standard clock having a drift that would need to be corrected, which would then need to be propagated to all the other clocks, which would introduce more errors due to the time taken to deliver the information. It would also cause a situation where during the synchronization after an offset adjustment, all the clocks would disagree with the central clock until the correct time could be carried around.
The time standard could be carried around by telegraph, by sending a synchronization message, but it too would have a propagation delay and timing error as stations would need to relay the message manually beyond the distance the signals would carry. That’s why it would have been more appropriate to establish a local time standard with accuracy, and then note down the relative difference between the stations – and calibrate each watch to be precise AND accurate according to the local time, and then adjusted by the known offset from central time.
You too confuse precision with accuracy. You can’t establish accuracy by comparing the clocks because all of them can run fast or slow – you can only tell if they’re all running at the same precise rate, but not whether they’re all accurate.
The issue for railroads is that as the central clock runs off, it needs to be adjusted periodically, and this offset then needs to be propagated to all the other clocks which takes time and introduces more errors the further you get from the central office. Hence, local standards for accuracy (celestial time) would be used and a known offset between the stations added to the results, establishing both accuracy AND precision to within the established standard.
Otherwise, sending a telegram from New York to San Fransisco to say “it is now 12:00” through relay stations along the way, or relaying an actual watch along the railway itself to all the stations, would accumulate too much random errors to be useful.
And back in the days of railroads and pocket watches, the need for accurate time led to the pocket watches of the day having the lever you had to unscrew the face glass to access, and you would pull lever out and set the time with the crown. The railroad watches did this to make it impossible to accidentally change the time when winding the watch.
With a sundial, ha ha.
That wouldn’t be all bad, considering the rotation of the earth only varies 0.1 milliseconds over a week.
A sundial can be made very accurate with a finely ground curved mirror that amplifies the difference in angle you get out of a sunbeam passing through a narrow slit.
Of course, if you want to take the human element out of the equation, what you do is put a magnifying glass in front of a sheet of copper, and a tiny tiny hole in the sheet. On the opposite side you put a single strand of hair and aim the contraption at the midday sun.
The hair will burn through the instant the focal spot shines through the hole, to within milliseconds. If then you have a watch placed opposite to a camera, you can use the hair trigger to photograph the dials and see what difference it has made a week later. That way any watch can be measured to very high degree of accuracy.
It all gets a bit Heisenberg though, sundials can be super accurate provided you know your exact longitude… how do you get your longitude, first, use a super accurate timepiece…
There is a sundial in the UAE I think that shows whole minutes in real time, but its huge- size of a building.
I like Luke’s idea of a hair trigger a lot- but substite nitinol wire, or better yet- use a photo diode to send current trigger to camera shutter. Maybe directed through a dark box underneath with mirrors to guide light through a large sweeping path before the trigger- giving microsecond solar arc resolution for the suns path.
Would have to correct for equation of time at a specific GPS coordinate though- but id kill to see a build like that
>” sundials can be super accurate provided you know your exact longitude”
It gets hairy when you want to know exact time at arbitrary points during the day, but if you only want to know when it’s noon, all you have to do is align the sundial with the earth’s axis of rotation by pointing it at the pole star.
>”but substite nitinol wire”
It’s too slow, and depends on the ambient temperature and air currents too much. This is a question in terms of 18th century tech – no photodiodes either.
More to the point – this isn’t a question of telling the exact time. This is a question of telling when a certain amount of time has passed a week or any number of days later.
With sundials, the noon is always when the sun crosses the plane defined by the axis of the earth and the zenith vector. The zenith vector you get with a simple plumb bob, and the axis of the earth by looking at the stars. Once you’ve established that plane, you can align your sundial so that the sunbeam shines through your aperture with only milliseconds of difference over a period of several weeks.
That is plenty to establish a standard for watches with precision of seconds per week.
Not a sundial, but with a meridian circle. A meridian circle is a special type of telescope that can only pivot along a north-south line, and has a hairline in the scope so that it is possible to observe the transit of a given star with extreme precision.
Sidereal time is determined by the stars. Any particular star will always transit at the same local sidereal time every day, and it’s easy to make a table of local sidereal transit times for many different stars. There’s also a fixed relationship between local mean solar time and local sidereal time (there is 1 more sidereal day than solar day in a year). So given the date and sidereal time of a stellar transit, you can compute/lookup the local mean solar time of that transit. This means that you can, any night that you can measure a transit, you can get an exact time fix, limited only by the accuracy and precision of your tables and calculations.
Meridian circles can be set up anywhere there is available sky, so anyplace which could afford an observatory and wanted one could have their own precise, accurate, time standard.
Historically, this was used to check the accuracy and precision of the best standards clocks of the day. If a particular transit was known to happen at 10:23:13 on one night, and the standard clock being monitored read 10:23:45, then the standard clock is 32 seconds fast. If a month later, another reading shows the clock to be 33 seconds fast, then the drift of the clock is 1 second/month. If, a year later, the drift is 1.5 seconds/month, then the drift of the drift is 0.5 s/mo/year. Computing the accumulated error at any given time, then using that to adjust the indicated time on the clock to the current time is more accurate and precise than adjusting the standard clock — which could take weeks for the drift to settle down after adjustment.
I don’t think that’s right. According to Wikipedia:
“maximum variation of 30 seconds (approximately 4 seconds daily) per weekly check” (https://en.wikipedia.org/wiki/Railroad_chronometer)
The Accutron was advertised as being accurate to 2s per day (Revolution in Time by David Landes),
“If you think you know about flip flops, by the way, you may find the end of the second a bit surprising, and quite the unusual use of flip flops.”
Umm… what? Are binary counters unusual now? I thought they were pretty common…
It was the literal use of flip flops the shoes.
Why only quartz is used in watches? As we know that other minerals like topaz, tourmaline also exhibits piezoelectric effect, why can’t we use these minerals in watches instead of quartz?