Monday | 24 October 1927 | Brussels
While the official title of the 5th Solvay conference was “on Electrons and Photons”, it was abundantly clear amongst the guests that the presentations would center on the new theory of quantum mechanics. [Planck], [Einstein], [Bohr], [de Broglie], [Schrodinger], [Heisenberg] and many other giants of the time would be in attendance. Just a month earlier, [Niels Bohr] had revealed his idea of complementarity to fellow physicists at the Instituto Carducci, which lay just off the shores of Lake Como in Italy.
The theory suggested that subatomic particles and waves are actually two sides of a single ‘quantum’ coin. Whichever properties it would take on, be it wave or particle, would be dependent upon what the curious scientist was looking for. And asking what that “wave/particle” object is while not looking for it is meaningless. Not surprisingly, the theory was greeted with mixed reception by those who were there, but most were distracted by the bigwig who was not there – [Albert Einstein]. He couldn’t make it due to illness, but all were eager to hear his thoughts on [Bohr’s] somewhat radical theory. After all, it was he who introduced the particle nature of light in his 1905 paper on the photoelectric effect, revealing light could be thought of as particles called photons. [Bohr’s] theory reconciled [Einstein’s] photoelectric effect theory with the classical understanding of the wave nature of light. One would think he would be thrilled with it. [Einstein], however, would have no part of [Bohr’s] theory, and would spend the rest of his life trying to disprove it.
Complementarity – Wave , Particle or both?

E = hv
Where E is the energy of the light quanta, h is Planck’s constant and v is the frequency of the light. The most important item to consider here is this light quanta, later to be called a photon. It is treated as a particle. Now, if you’re not scratching your head in confusion right about now, you haven’t been paying attention. How can light be a wave and a particle? Join me after the jump and we’ll travel further down this physics rabbit hole.
The Other Side of the Quantum Coin
While the wave – particle duality of light was busy mystifying the world’s smartest physicists, Prince [Louis de

Broglie] of France had an odd idea. If an electromagnetic wave can have a particle nature, could particles have a wave nature? In 1923 [de Broglie] introduced academia to his hypothesis with his PhD thesis. He would be awarded the Nobel Prize in Physics in 1929, two years after his thesis was verified by an English physicist by the name of G.P. Thompson. Thompson fired X-rays and electrons, two entities whose wave and particle nature were obvious, at a thin sheet of aluminum foil with a teeny tiny hole in it. The results of the X-rays emanating from the tiny hole showed what classical physics would predict what a wave would show – a diffraction pattern. The stream of electrons showed the same diffraction pattern, proving the wave-like nature of the electron in accordance with [de Broglie’s] hypothesis. The fact that a particle can display wave-like properties puts into question the ability to know precisely where it is in space and time.
The End of Determinism
So how does one go about trying to locate an electron anyway? According to [de Broglie’s] hypothesis, the smaller the dimensions of the particle, the more wave-like it becomes. Electrons are so small, that saying one is at a particular point in space at a particular point in time is not really possible. It’s too wave-like to make this type of observation. There are numerous ways to illustrate this idea, better known as [Heisenberg’s] uncertainty principle. My favorite, and I think the easiest, way to understand this is mathematically. Consider the following super advanced complex quantum physics equation:
xy = z
Let 'x' equal momentum
Let 'y' equal position
Let 'z' equal the constant of h/4pi. Where 'h' is [Planck's] constant.
It should be easy to see that x and y are inversely proportional. As one goes up, the other goes down. In other words, as you increase the value of the momentum of a particle (x), the accuracy of the position of that particle (y) decreases. And vice versa. This is the heart of [Heisenberg’s] uncertainty principle. You can’t know both the location and momentum of a particle at the same time. The more accurate you make one, the less accurate you make the other.
The Copenhagen Interpretation
[Niels Bohr’s] complementarity theory together with [Heisenberg’s] uncertainty principle makes up what is known as the Copenhagen Interpretation of quantum mechanics. This theory, while still contested to an extent, is the most widely held view of the nature of reality to date. To put it in the most simplified terminology – It’s not possible to know if our subject of investigation is a particle or wave, just like it’s not possible to know its location and momentum. You can know one, but not the other. Neither can be known at the same time. There is an inherent uncertainty, an inherent randomness to our universe. It is ingrained in our very existence, and to deny it is futile.
Now that our little history lesson in quantum theory is done, we can get to the fun part of applying our new found knowledge to hacking! We now know that the electrons zooming around in our microprocessor have a wave like property. What does this mean? Where can we go with this? This will be the subject of next week’s installment of Quantum Mechanics in your Processor. Stay tuned!
















One way of thinking of wave/particle duality is to say the particle has x, y, z and w dimensions but you can only observe 3 dimensions. So when you observe the quantum particle one of the 4 dimensions collapses
Interesting perspective
could the w axis be the aether ?
aether doesn’t exist. Its existence have been ruled out by Einstein relativity theory. Also by the famous Michelson and Morley interferometer experiment: https://en.wikipedia.org/wiki/Michelson%E2%80%93Morley_experiment
“Aether” is fundamentally the idea that empty space has no properties, so it cannot carry any forces or fields. The Aether is stuff that fills all space and mediates forces similiarily to how ideal gasses transmit waves by collision of molecules, except in Aether it’s some fundamental particle that does only one thing like transmit magnetic force, or the weak nuclear force etc. etc. and in doing so it must aquire some very magical properties, such as:
” the mechanical qualities of the aether had become more and more magical: it had to be a fluid in order to fill space, but one that was millions of times more rigid than steel in order to support the high frequencies of light waves. It also had to be massless and without viscosity, otherwise it would visibly affect the orbits of planets. Additionally it appeared it had to be completely transparent, non-dispersive, incompressible, and continuous at a very small scale.”
So the problem with the Aether theory is that it’s basically an attempt to explain reality as a purely mechanical, but even on a philosophical point that would have required point entities described with infinite amount of information and other incredulities, so the whole idea was dropped.
Now if we rename it to ‘dark energy’ or ‘dark matter’ then suddenly magic is OK as a concept in science :)
Not at all. “Dark Matter” is just the name we use for “That stuff that we think must exist because we see something we can’t explain yet”.
If a ship is lost at sea, and we can’t explain why, it was taken by “The Kraken”. This is not an actual creature. It is just a name given to “The reason it happened, that we don’t understand yet”.
Does that make sense?
(note: if you use a term for long enough, it actually does start to have a specific meaning whick can branch outside of the original use. The Kraken, and Gremlins are good examples of this.)
Thanks!! This is one of the clearest explanations I have read on quantum crazyness!
@ Will Sweatman,
Not [de Broigle], neither [de Brogile] but [de Broglie].
Thanks! I think I’ve got them all corrected.
Why are you writing “[de Broglie]”? Which roughly translated is just “from Broglie”, which is obviously not a noun. And what are the “[ ]”‘s for? He is Louis-Victor-Pierre-Raymond, 7th duc de Broglie, or more commonly just “Louis de Broglie”. And for completeness; Broglie is pronounced (simplistically) as “Broy” where the “oy” is as in “boy”.
“Thompson fired X-rays and electrons, two entities whose wave and particle nature were obvious”
…
“The stream of electrons showed the same diffraction pattern, proving the wave-like nature of the electron”
Please fix the logic error
you’re missing the obvious point.
“””
xy = z
Let ‘x’ equal momentum
Let ‘y’ equal position
Let ‘z’ equal the constant of h/4pi. Where ‘h’ is [Planck’s] constant.
It should be easy to see that x and y are inversely proportional. As one goes up, the other goes down. In other words, as you increase the value of the momentum of a particle (x), the accuracy of the position of that particle (y) decreases.
“””
Not the way most people would interpret “momentum” and “position”. The inverse relation works on the values, not for their precision.
Maybe you can elaborate and explain why it actually makes this difference?
P.S. I’ve always wondered how HUP works, but most “simple” explanations out there sound incorrect or circular.
I should probably just go ask a physics professor…
Yeah, I had the same thought. The way I interpret the xy = z thing is if momentum goes up the position goes down and vica versa. So, the position changes not the accuracy of the position. I am probably not seeing something…
The xy = z is not the actual equation. There are several analogies out there that give a simplified explanation of the uncertainty principle, this was the one I chose. If comparing it to the actual equation, the x and y would symbolize the uncertainty of measuring position and momentum. And the equal sign would need to be changed to a greater than or equal to. But this gets confusing and beyond the scope of the article. The point to take away is the more precise you make one value, the less precise you make the other.
I think that what you (and the author, maybe) are not seeing is that x and y stand for the accuracy of the measurements, not the values themselves. If it were the values, just changing the position of an object would change its speed (momentum), and clearly this is not so.
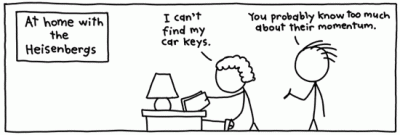
The xkcd drawing explain this phenomenon better: one stick figure doesn’t know exactly the position of the keys, ergo maybe he knows the keys speed with too much precision.
Here’s a easy way I find to think of it. I’m afraid I can’t speak to it’s accuracy.
Lets through it on it’s head, and look at the position and momentum of the particle as a wave.
Say we do a FT on the wave and get a single pure frequency (absolute knowlegde of it’s frequency and there fore it’s momentum) we must there for have many cycles in the time domain (infinite in fact). and therefore no idea where it is.
Lets tighten up the single until it’s a small wave packet and do an FT and now we have various frequencies popping up as though the single now has various momentums.
Tighten the signal fully to a spike and the FT will spew out infinite frequencies.
That is to say the more sure you can be about a waves frequency the less sure you can be about it’s location (in effect the surer we are about it’s frequency the more spread out the wave must be).
Ha! Just watched the video below and I must have totally ripped this explanation off from someone. Hilarious. I actually thought I’d thought it up myself.
I am not as smart as I believed!
“To put it in the most simplified terminology – It’s not possible to know if our subject of investigation is a particle or wave, just like it’s not possible to know its location and momentum. You can know one, but not the other. Neither can be known at the same time.”
Doesn’t math seem to say that you can know both, just not very precisely??
http://imgs.xkcd.com/comics/location_sharing.png
I get a real chuckle out of this cartoon.
Me, too :)
I’d have the opposite view though, I’d sooner have them get my momentum than my location.
One question that I have is whether the “uncertainty” applies to the ACTUAL properties, or only to the OBSERVABLE properties. That is, can a particle HAVE a precise momentum and velocity, even though these cannot both be observed with precision? Web sites that I have found seem to disagree on this. Does any one know Heisenberg’s, Bohr’s and/or Einstein’s thoughts about this (and no fair saying none of them were certain!)?
The Copenhagen Interpretation of QM, trumpeted by [Bohr] and [Heisenberg], says it applies to the actual properties. Observation determines reality. [Einstein] did not agree with this view.
This is all the debate about quantum physics. Is There an objective reality or reality is only what you observe. What Bohr says is that reality is what one observe and nothing more. But Einstein defended the objective reality.
This debate is not over yet and maybe will never be.
@Jacques1956
You (and those who are having the debate you mentioned) are confusing the Uncertainty Principal with the Observer Effect.
The Uncertainty Principal is a “…mathematical inequality asserting a fundamental limit to the precision with which certain pairs of physical properties of a particle…can be known simultaneously”
While the Observer Effect “…notes that measurements of certain systems cannot be made without affecting the systems”.
The key word with the Uncertainty Principal is KNOWN. This does not mean that particles do not HAVE specific physical properties. It means that we cannot use math to determine all of a particles properties if we don’t already KNOW all of the properties. This is unintuitive because math is normally the tool we use to find such missing information.
This is further complicated because the things we are studying cannot be directly observed, which is where the Observer Effect starts to cause problems. If we want to determine a property of a particle, we need to measure it, which often involves putting something in it’s way and seeing what happens when the particle smashes into it.
Imagine if the only way to measure the speed of a train was to build a brick wall on the tracks and then look at the pattern the bricks and train made after the train plowed into the wall. Even if the train stayed on the tracks and kept going, you would only know how fast it was going BEFORE it hit the wall. You would have to build another wall for it to smash into if you wanted to know it’s new speed.
Since the Observer Effect and the Uncertainty Principal both affect our ability to determine the properties of particles, they are often used together. This is probably where the confusion comes from.
@Robert Piston
Particles have real properties. Even when we are not looking at them.
The Uncertainty Principal means that PREDICTIONS of the results of an interaction can only be so precise.
The Observer Effect means that we cannot know the current properties of a particle, only past properties, which were changed when we checked them.
Of course, this all depends on you being a realist I suppose.
Some people really DO believe that Observation = Existence.
I don’t think they are. Actually the argument is made that they do not have real properties until measured. That is to say if you pin down the position there is no longer a certain momentum to the particle because there is no certain property.
A simple experiment with thinner and thinner tall slots in a opaque sheet and a coherent light source( x dimention is thin, y dimension is tall and no z dimention it’s a thin sheet).
What happens? The beam narrows then begins to spread?!
wave theory says it is diffracting.
Whats happening to the particles? Why did they change direction? (spreading means the angle of the photons relative to the sheet are no longer 90degrees)
Well if we use a narrow slow we begin to gain a lot of information about the photo in the position x direction (not the y or z) and suddenly the momentum in the x direction becomes unpredictable.
I once encountered a notion that basically said: a wave needs a medium to travel through (think waves in water, or audio waves in air), as it is basically a displacement occurring in that medium. The dual wave/particle nature of light can be thought of as the photon particles being the physical medium that the waves travel through.
Now, I have no idea if that is actually a useful view or not. But it sounds nice. :) I don’t remember where I heard or read that, though. Has anyone else encountered that explanation?
I’ve read several statements from [Bohr] saying something like “It’s temping to try to develop a mental picture of a photon or any particle in a predetermined state independent of measurement. But this is not helpful and often misleading.”
I’d like a source for that : )
this doesn’t match the results of the double slit experiment, especially re: sending particles through the slits one by one.
@Will Sweatman
“…if you’re not scratching your head in confusion right about now, you haven’t been paying attention. How can light be a wave and a particle”?
No. The confusion exists because light is NOT “Both a [Wave] and a [Particle]”. The problem here is improper use of words/ideas.
[Waves] and [Particles] are two clearly defined descriptions of specific things. [Waves] are not [Waves] because we define them as such. They already exist. We choose to call them [Waves] because we observe their properties and it helps us to group things by their similarities. The same applies to [Particles].
Therefore, light is “neither a [Wave] nor a [Particle], but sometimes it acts similar to a [Wave] or a [Particle] in certain situations”.
Here is a simple (if silly) example:
[Blueberries] are small berries that grow on bushes and are blue.
[Red Meat] is muscle that is red when uncooked and not white when cooked
If we find [Mystery Meat], which is blue colored protein that grows on a bush and does not turn white when cooked, it is not “Both a [Blueberry] and [Red Meat]” because that would be silly. [Mystery Meat] does not meet the definition of [Blueberries] or [Red Meat] so it is something else.
Light works the same way.
It may be useful to sometimes think of [Mystery Meat] as [Red Meat] or [Blueberries] based on our situation. If we are looking for a material to extract blue coloring from we might treat [Mystery Meat] as we would [Blueberries]. If we need meat to make hamburgers, [Mystery Meat] or [Red Meat] might be equally suited. But, there will be some times when it is not appropriate to think of [Mystery Meat] as [Blueberries] or [Red Meat] because it does not fully fit into either definition.
Similarly, it may sometimes be useful for us to think of light as a [Wave] or a [Particle], because it may help us to understand a particular interaction. However, we must recognize that there are other times when it is not appropriate because light will behave differently.
It is important to remember that all of these things exist independent of us. Naming things and grouping them by similar properties are just tools that can help us understand our universe. Using the right tool for the job, and using it correctly, is important.
TLDR;
Light is NOT “Both a [Wave] and a [Particle]”.
Light is “neither a [Wave] nor a [Particle], but sometimes it acts similar to a [Wave] or a [Particle] in certain situations”.
The distinction is important, because we use words to convey ideas. Using the wrong words conveys the wrong ideas.
Note:
I realize that the convention here is to use [square braces] around names. However, since we cannot bold or italicize in our posts, I have chosen to use them around terms to draw attention the reader’s attention because I feel it helps clarify the statements.
Ian, you should definitely write more about how you see the world.
Your words here are one of the most deep and clear interpretation I could read in decades.
Thank you very much!
You can actually bold in posts, use.XHTML.
XHTML: You can use these tags: <a href=”” title=”” rel=””> <abbr title=””> <acronym title=””> <b> <blockquote cite=””> <cite> <code> <pre> <del datetime=””> <em> <i> <q cite=””> <strike> <strong>
As for your post, seems indeed a better way of putting it, but of course this isn’t meant to be the definite textbook on quantum physics anyway, but more an attempt to explain it simply.
Thanks for providing this, good to see this kind of input. Shame it is not at the top.
I agree with RoGeorge, your expressiveness is remarkable. If you wanted to start a (quantum-)physics blog, I’d gladly read it.
Its also worth noting that the Copenhagen interpretation relies on the notion of an observer.
There is another interpretation – equally accurate – which tries to explain this in another way.
Its called consistent histories. They are entirely equivalent.
Just as @Ian indicates above – use of terms like wave and particle, when the thing is neither, can lead to confusion. So can the term observer. I’d encourage you to lookat/buy the (no mathmatical proofs) book called Quantum Philosphy by Omnes http://amzn.to/1Jl5ZXX or read about this approach and decoherence here: http://plato.stanford.edu/entries/qm-consistent-histories/ or on wikipedia of course :)
Please correct “visa verse”. It should be “vice versa”, from Latin.
“This theory, while still contested to an extent, is the most widely held view of the nature of reality to date. ” This is true but a little misleading. According to one poll on Sean Carrol’s blog, only 42% favor the Copenhagen interpretation. A plurality but definitely not the majority.
http://www.preposterousuniverse.com/blog/2013/01/17/the-most-embarrassing-graph-in-modern-physics/
To me the Copenhagen interpretation is kind of a cop out. It’s equivalent to saying “shut up and calculate”. The thing is that this isn’t the first time we have been in a situation like this. For a long time there were many valid ways of interpreting the solar system. The Greeks believed in a geocentric system with additional epicycles to account for discrepancies. Today this is considered completely wrong, however the Greeks were able to get very accurate calculations and predictions with the geocentric model. There is no doubt that quantum mechanics can produce extremely accurate predictions, however I wouldn’t be surprised if the Copenhagen interpretation is closer to accepting a geocentric model and future scientists will look back at this period with disbelief that we missed an obvious realist interpretation.
Here’s a short version that I hopes help clear up Heisenberg’s uncertainty principle a bit because many seem confused. This is a bit oversimplified but here’s a stab at it.
Where is an electrons position and velocity?:
1.) Lets shine some light on it to observe what happens:
2.)To bounce light off of it we need to know that we are hitting it with a photon. Where is the photon I want to hit it with? It is most likely found at the peak of the wave. The finer my peak the better odds of fiding it.
More energy = “vibrates faster” (Higher frequency) = Finer peak
Less Energy = “Vibrates slower” (Lower Frequency) = broader peak
3a.)If we hit it with a closely spaced(High frequency)[photon] wave we can see its position but now that we have hit it with a photon its momentum has changed.
The greater our precision(higher energy = narrower wave/finer peak) the more energy required and the more energy in the photon the more it changes the momentum of the electron.
3b.)If we hit it with a widely spaced (Low frequency)[photon] wave we can see the momentum of the electron because it has received very little energy from our measurement but now we are very unsure of where our photon is likely to be because its peak is very wide and thus there are lots of places it can be.
Heisenberg himself tried to make this easier to understand in his thought experiment “Heisenberg’s Microscope”; This guys explains it surprisingly well:
https://www.youtube.com/watch?v=dgoA_jmGIcA