Einstein referred to her as the most important woman in the history of mathematics. Her theorem has been recognized as “one of the most important mathematical theorems ever proved in guiding the development of modern physics.” Yet many people haven’t the slightest clue of who this woman was, or what she did that was so significant to our understanding of how our world works. If you count yourself as one of those who have never heard of Emmy Noether and wish to enlighten yourself, please read on. I can only hope I do her memory justice. Not just by telling you who she was, but by also giving you an understanding of how her insight led to the coming together of symmetry and quantum theory, pointing academia’s arrow toward quantum electrodynamics.

Being a female in Germany in the late 1800s was not easy. She wasn’t allowed to register for math classes. Fortunately, her father happened to be a math professor, which allowed her to sit in on many of his classes. She took one of his final exams in 1904 and did so well that she was granted a bachelors degree. This allowed her to “officially” register in a math graduate program. Three years later, she earned one of the first PhD’s given to a woman in Germany. She was just 25 years old.
1907 was a very exciting time in theoretical physics, as scientists were hot on the heels of figuring out how light and atoms interact with each other. Emmy wanted in on the fun, but being a woman made this difficult. She wasn’t allowed to hold a teaching position, so she worked as an unpaid assistant, surviving on a small inheritance and under-the-table money that she earned sitting in for male professors when they were unable to teach. She was still able to do what professors are supposed to do, however – write papers. In 1916, she would pen the theorem that would have her rubbing shoulders with the other physics and mathematical giants of the era.
Noether’s Theorem – The Basics
Emmy Noether’s Theorem seems simple on the onset, but holds a fundamental truth that explains the fabric of our reality. It goes something like this:
For every symmetry, there is a corresponding conservation law.
We all have heard of laws such as Newton’s first law of motion, which is about the conservation of momentum. And the first law of thermodynamics, which is about the conservation of energy. Noether’s theorem tells us that there must be some type of symmetry that is related to these conservation laws. Before we get into the meaning, we must first understand a little known subject called The Principle of Least Action.
The Universe is Lazy
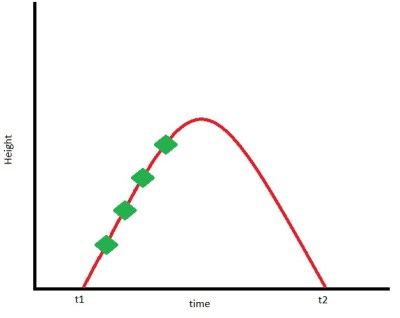 I would wager a few Raspberry Pi Zeros that many of you already have an intuitive grasp of this principle, even if you’ve never heard of it before now. The principle of least action basically says that the universe has figured out the easiest way possible to get something done. Mathematically, it’s the sum over time of kinetic energy minus potential energy as the action occurs. Let us imagine that you’re trying to program an STM32 Discovery eval board in GCC. After about the 6,000th try, you toss the POS across the room and grab your trusty Uno. The graph depicts the STM32 moving through time and space.
I would wager a few Raspberry Pi Zeros that many of you already have an intuitive grasp of this principle, even if you’ve never heard of it before now. The principle of least action basically says that the universe has figured out the easiest way possible to get something done. Mathematically, it’s the sum over time of kinetic energy minus potential energy as the action occurs. Let us imagine that you’re trying to program an STM32 Discovery eval board in GCC. After about the 6,000th try, you toss the POS across the room and grab your trusty Uno. The graph depicts the STM32 moving through time and space.
The green points represent particular points of how how high the STM32 is at a given point in time. Note that there are no values for height and time – this example is meant to explain a principle. We can say that at these points (and all points along the curve), the SMT32 has both kinetic and potential energies. Let us call the kinetic energy (kt) and the potential energy (pt). The ‘t‘ subscript is for time, as both the energies are functions of time. The action for each point will be called s, and can be calculated as:
However, action is the total sum of the difference of energies at each point between t1 and t2. If you’ve read my integral post, you will know that we need to integrate in order to calculate the total action.
Now before you get your jumper wires in a bunch, all that is saying is that we’re taking the difference in potential (p) and kinetic (k) energies at each point along the curve between t1 and t2, and we’re adding them together. The elongated S symbol means a sum, and the (dt) means as it changes over time. The path that the STM32 will take will be the path where the action S is at its minimum value. Check out the video in the source section below if you’re confused. It’s only 10 minutes and goes into this concept in easy to follow details.
Noether’s Theorem – The Details
 Noether’s theorem is based upon a mathematical proof. It’s not a theory. Her proof can be applied to physics to develop theories, however. Now that we know what the principle of least action is, we can do just this.
Noether’s theorem is based upon a mathematical proof. It’s not a theory. Her proof can be applied to physics to develop theories, however. Now that we know what the principle of least action is, we can do just this.
Any law of nature can be traced back to a symmetry and the least action principle. Let’s consider two very simple examples – Newton’s first law of motion and the first law of thermodynamics.
Conservation of Momentum
Space has what is known as translational symmetry. That’s just fancy-pants talk for saying that what you do in one point in space is the same as what you do in another point in space. It doesn’t matter what hacker space you throw your STM32, it will act the same at all hacker spaces on earth. Space itself provides the symmetry. And because the principle of least action applies, you have a natural law – the first law of motion.
Conservation of Energy
Time has the same translational symmetry as space does. If I toss the STM32 now, and toss it tomorrow, it will act the same. It doesn’t matter what point in time I toss it, the results will always be the same. Thus energy is conserved between different points in time. Time is our symmetry, and the 1st law of thermodynamics is the result.
Now, I realize these examples might seem a bit useless. But when you dig a bit deeper, things get interesting. Electrical charge is also conserved. Noether says there must then be some type of symmetry involved. What do you suppose that symmetry might be? Keep following that rabbit hole, and you’ll end up face to face with QED. We’ll get there in a future article, so for now just keep Noether’s Theorem in mind.
Sources
Physics Helps, The principle of least action, video link.
Ransom Stephens, Ph.D., Emmy Noether and The Fabric of Reality, video link















Very good, thanks for doing this.
Very good article – I look forwards to the rest of the series
I thought this would be about Noether’s Clock!
It really was a stunning insight.
Do we have a complete one-to-one mapping between every known symmetry and every known conservation law?
More or less.
It’s actually more straightforward than you think: in general, if you postulate something being conserved (which usually gets called a “conserved current”), you’re kindof saying “the derivative of this is zero,” which means if you just imagine integrating that object, you get back an integration constant which can be freely changed and none of the physics changes. That symmetry that changes that constant is the symmetry.
That being said, the “classic” example of Noether’s theorem applies instinctively only to continuous symmetries – like rotation, translation, boosts, etc. Ones that you can move the system by small, medium, large amounts. You can also have discrete symmetries: like time reversal or parity, for instance, where you have different states of the system that are identical, but finitely many of them. For discrete symmetries, it’s a little different, although there’s something similar that would apply there, just not Noether’s theorem.
But for all continuous symmetries, yeah, there’s a complete one-to-one mapping. It’s just math to get from one to the other.
Yes. Well we should. Its obvious once you see it in action.
http://www.lecture-notes.co.uk/susskind/classical-mechanics/lecture-4/symmetry-and-conservation/
That link also has a link to susskind giving that lecture on YouTube.
Put simply a symmetry means there is a coordinate transformation which doesn’t make a change in the action.
For example adding a small amount epsilon to the t coordinate.
The change in action is zero so a quantity must be conserved this is the brother charge. And for time that is energy.
I will give you another example -just for fun- rigid bodies
Conserved quantities:
For an ideal rigid body (for as long as it can be called one), all its particles maintain the same pairwise distance.
Symmetry:
Identical translation and rotation of the particles of the rigid body with respect to their original position.
“Time has the same translational symmetry as space does. If I toss the STM32 now, and toss it tomorrow, it will act the same. It doesn’t matter what point in time I toss it, the results will always be the same. Thus energy is conserved between different points in time. Time is our symmetry, and the 1st law of thermodynamics is the result.”
There’s a funny thing about this.
It’s wrong.
It’s actually not the Universe that’s conserving energy. It’s physicists. The Universe isn’t lazy – physicists are. Writing down time-dependent laws of physics is annoying, and so they don’t do it. And in general, the Universe *seems* time invariant, so it makes sense to just say “no, screw it, it’s *totally* time invariant,” and ignore the difference.
But on a cosmological scale… it’s not time invariant. Not in the tiniest bit. We know this. There’s a big giant fireball remnant in the sky shifted to microwave frequencies that tells us this all the time. “Something happened” in the Universe at inflation times, and *everyone in the Universe* can agree on when that happened.
So, if the Universe *isn’t* time invariant on cosmological timescales, by Noether’s theorem, it doesn’t conserve energy. And, well, it doesn’t. (You can extend the same arguments to angular momentum/momentum as well – inflation provided a preferred reference frame, and so all of the nice happy things taught in high school physics class are, on a global scale, bunk).
And when you get your Planed Express ship it will matter a lot! Bunk indeed. Pollywash I say! Get a job. I’m taking a nap.
This isn’t actually particularly controversial, or even difficult to see. The Universe’s expansion is accelerating. That can’t happen unless you’re feeding in energy, and unless we find evidence that it will ever slow down, there’s no advantage to attempting to add fields to conserve energy. It’s similar to when inflation occurred – that had to require energy, and so physicists invented a field to conserve it.
This also isn’t related to “free energy” or anything like that. On non-cosmological scales, energy looks pretty well perfectly conserved, so unless you figure out how to manipulate whatever caused inflation, or whatever is driving dark energy, it’s not going to help you at all.
This is the direction my mind was going.
Can we assume symmetry applies in an expanding universe?
Is the assumption that dark energy exists our attempt to force symmetry on an asymmetrical universe? Symmetry would assume a stable system, where any system that has a starting point of existence at one point is not symmetric.
“Is the assumption that dark energy exists our attempt to force symmetry on an asymmetrical universe?”
Well… maybe. If dark energy is just the simple fact that empty space has negative pressure, then… yes, it is. With that fact, space is inherently unstable – you create a tiny bit of it, and boom, it expands forever. So the idea of time or space symmetry in that setup is nonsense. Enough matter could stabilize it, obviously, but the observational data currently says “no, it’s not stable.”
But that’s different than saying symmetry doesn’t apply in an *expanding* Universe. You can still have conservation of energy with an expanding Universe – it just has to eventually become static. In that case, there still was obviously something that was *asymmetric* to cause the expansion in the first place (e.g. something like spontaneous symmetry breaking) but symmetry can still exist, overall.
Dark energy screws all that up, though. Now you’ve got no idea what the hell is going on.
I misspelled Planet Express or you would have known I was referring to Professor Farnsworth’s drive system that causes the universe to move while the Planet Express ship stays still.
You simply assume that a Big Bang theory is equivalent to a Big Bang, for which there is zero evidence, As for inflation theory, it is self-inconsistent in claims made about it. First, once started, inflation is eternal eternal. Second, eternal inflation directly implies a mulitverse in which bubble universes pop out of the inflation field with any properties whatsoever with no limits. Conseqjuently, no set of observations can ever falsify inflation theory: if a classical, textbook model of inflation is inconsistent with Planck13 observations, toss the model and make another that is tuned to observations. There are well over 100 models of inflation, each tuned to observations. Linde, one of the 3 founders of inflation theory, actually brags that the theory can’t be falsified, then makes the evident logical fallacy of claiming that “predictions” of inflation theory have been repeatedly confirmed! The varous MODELS of inflation theory make predictions that can be and have been falsified, but the fact that inflation theory itself with its anything-goes universes cannot be falsified necessarily means that it makes no predictions. And NO, not every theoretical physicists in world believes that the universe had a beginning with a singularity about 13.8 billion years ago in which general relativity (GR) breaks down and spews meaningless infinities. No one can believe that the universe began as a mathematical point because quantum theory forbids this and also forbids any length smaller than Planck length. As far as anyone knows or can even investigate mathematically, neither at the beginning of expansion of spacetime nor in the center of black holes, there is no reason to assume that a mathematical singularity implies a physical one. Such a singularity normally means the theory requires modification. Given the breakdown of GR with densities far greater than any we can hope to create in the foreseeable future, we have no reason to assume that the field equations of this classical theory are applicable to densities at which quantum effects become large. Believe if you wish in a Big Bang, a physical singularity, and in inflation, which loses its status as a scientific theory when it cannot be falsified, which also means it does not make predictions. You might as well include the tooth fairy and Santa Clause.
The funny thing is that not long ago you would never hear of Noether’s Theorem, even in a physics PhD program, except by a lucky accident while prowling through the stacks in the library. Now it pops up in answers and explanations on the physics stack exchange all the time, and even HaD. It is quite powerful and fits in with other over-arching insights like the Hamiltonian and Lagrangian, which are way cool.
I majored physics as an undergrad. We covered Noether’s theorem in “Classical Mechanics” (3rd year, 23 years ago). As you say, it’s important in the context of Hamilton’s mechanics.
That is interesting.1993? It is good to know these things are more widespread. I guess for me “not long ago” is now more like 40 years! The only person I knew who covered this in a formal course, got it as a grad student member of the theory group at Stanford under Leonard Schiff (who passed away shortly after).
This is in a nutshell what Boscovich theory postulated 150 years before that (1745).
Dear Will Sweatman,
Sorry I need to criticize your tutorial regarding group theory again or whatever this tutorial may cover… The whole tutorial lacks motivation, proper notations, definitions and historical correctness. The definition of an integral is more than incomplete. You jump from A to Z. Do not write a tutorial without having a deep knowledge about the things you want to teach. I would like to offer you to proof read this article series prior publishing.
I have to agree to some degree.
“The elongated S symbol means a sum” is what set me off.
I’m not sure if my understanding of the English language is of(f) or the sentence is missing something like “areal sum over the area between…” (“size of the area between …” is even better).
Even the mouse-over tells me it’s an int(egral).
On the other hand – this is not an Scientific article written to be published in a paper.
Hi Markus Bindhammer,
My goal in STEM articles is to explain the subject matter in a way that someone who has never heard of it before can actually understand, and do so in an entertaining fashion. I strongly disagree with the notion that one needs a “deep knowledge” of a subject to understand and teach the basics. In fact, being a subject matter expert can often hinder a person’s ability to explain it to a n0ob. STEM Wikis are a great example of this.
I will continue to write STEM articles for dummies like myself. There are over 9000 people out there who are interested in these subjects, but lack the background to understand them. I am their only hope.
Will, I love the accessibility of your articles and many of us find the broad strokes of physics interesting without the need for “deep understanding” of the technical high level math. Not that deep understanding isn’t important, but explaining complex concepts in a way the general public can digest is actually important. Everybody can’t be a mathematician or PhD level physicist. Keep it up.
Perhaps in a future article you could explain the symmetry that leads to conservation of Baryon number, lepton number or strangeness. And tell us the conserved entity associated with local gauge symmetry.
Also, if you are really going to tell us the symmetry that causes conservation of charge, note that the universe does not obey charge conjugate symmetry (swap pos charges with neg charges), nor does it observe Charge-Parity symmetry (swap pos charges with neg charges, and also mirror image the setup).
Noether’s theory assumes a smooth manifold, which means measures (such as position) can have infinite precision. In other words, position is not quantized, which implies the universe is not computable.
To put this more clearly, assume you are writing a computer program to simulate the universe. How wide are the floating point numbers that represent position? If Noether’s theorem is correct, you need infinite precision to represent position… but an infinitely long number cannot be calculated in a finite amount of time, so no computer can simulate the universe if Noether’s theorem is true.
Entropy and energy are related through temperature. If Noether’s theorem is true, the position of any single particle would have infinite information, thus infinite entropy, and (if the temperature is non-zero) infinite energy.
Noether’s theorem is a fine example of mathematical understanding, but it was written before Shannon invented/discovered information theory. It is based on an assumption that further research indicates cannot be true.
It’s probably a bit like Newton’s laws of motion: true for most purposes, but doesn’t describe the complete picture.
By “true” I mean “true representation” of our actual universe. The theorem can be proven true mathematically, but it still may not be the correct model to use.
Charge conjugation symmetry (‘C’) isn’t conservation of charge. It’s a discrete symmetry involving taking all particles and flipping all charges. Conservation of charge says that the total charge of the system stays constant. These are different things.
Also your “discrete/continuous” argument doesn’t hold up. There’s a difference between the *math you use* to represent a system and the *information content* of the system itself.
You can’t store infinite information in the energy of a particle even if it *is* continuous, because localizing the energy of a particle to a specific value at a specific time is impossible – that’s Heisenberg uncertainty for you. Information is stored in the number of *states* of a system, not the specific values. Energy, position, momentum, etc. aren’t real fundamental things you can encode information into.
Fair enough, Will!
All these theories we have to make up for our inability to perceive that when we throw the item it is actually travelling in a straight line in space time. I blame gravity.
Well, sort of. If it is a point mass. Otherwise tidal forces prevent it from following a true free-float geodesic.
“Being a female in Germany in the late 1800s was not easy.”
Compared to women now, that is correct. Compared to men in Germany at the time, I strongly disagree. She was at least sheltered from being the human plow horse of society, and the cannon fodder of every ambitious politician.
Probably not.
There were no bachelors degrees in Germany before the Bologna declaration. What you call a bachelors degree in Emmy’s life is probably the Abitur which is comparable to a high school diploma and the french baccalauréat.
“””
For every symmetry, there is a corresponding conservation law.
We all have heard of laws such as Newton’s first law of motion, which is about the conservation of momentum. And the first law of thermodynamics, which is about the conservation of energy. Noether’s theorem tells us that there must be some type of symmetry that is related to these conservation laws.
“””
Technically, the theorem you’ve stated says nothing about whether a conservation law necessarily has an associated symmetry. (Compare: “All CEOs are rich. Therefore all rich people are CEOs”.)
I haven’t found any other statement of the theorem that includes the converse(“For every conservation law there is a corresponding symmetry”), but this article seems to exclusively use the theorem in this sense. Is there something I’m missing?
It’s stated this way in the article, but the theorem’s proof is reversible. A continuous symmetry produces a conserved current, and a conserved current generates a continuous symmetry.
Thanks. I did try to do some research of my own but most sources quickly got too advanced for me.
Does this mean I need to stop calling myself lazy, and start calling myself symmetrical?
Will:
“Let us call the kinetic energy (kt) and the potential energy (pt). The ‘t‘ subscript is for time, as both the energies are functions of time. The action for each point will be called s, and can be calculated as:”
That’s actually a quantity called the Lagrangian, not the action. The Lagrangian is basically the total energy of the system. Then the integral of the Lagrangian over time is the action, and the principle of least action says the system moves such that the action isn’t changing. So the ‘little s’ should be an L, and everything’s right.
Poor little STM32 board. People always fear what they don’t understand.
I’m with you on that Donno, stop mistreating these trustworthy, super easy to program stm’s !
Can I say the ball is tracking a straight line path in a one dimensional trajectory through space time.