Unless you are above a certain age, the only time you may have seen a slide rule (or a slip stick, as we sometimes called them) is in the movies. You might have missed it, but slide rules show up in Titanic, This Island Earth, and Apollo 13. If you are a fan of the original Star Trek, Mr. Spock was seen using Jeppesen CSG-1 and B-1 slide rules in several episodes. But there was a time that it was common to see an engineer with a stick hanging from his belt, instead of a calculator or a cell phone. A Pickett brand slide rule flew to the moon with the astronauts and a K&E made the atomic bomb possible.
Slide rules are a neat piece of math and history. They aren’t prone to destruction by EMP in the upcoming apocalypse (which may or may not include zombies). Like a lot of things in life, when it comes to slide rules bigger is definitely better, but before I tell you about the 5 foot slide rule in my collection, let’s talk about slide rules in general.
History of the Slide Rule
The Reverend [William Oughtred] (who probably introduced X as the multiplication symbol) developed the slide rule in the 17th century, so they’ve been around for a while and were standard issue for people serious about math until the early 1970s. Actually, doing math on a ruler wasn’t a new idea even then. [Edmund Gunter] devised a sector with slide rule-like scales, but you needed a separate set of dividers to solve problems with it. [Oughtred’s] device was a circular slide rule and one of his students, [Richard Delamain], also claimed to have invented the device. Both men accused the other of stealing it.
Scholars now think that both men developed the circular slide rule independently. [Delamain] was the first to publish although [Oughtred] apparently finished his device first. However, [Oughtred] definitely developed the straight slide rule around 1650.
The Theory Behind the Rule

Slide rules depend on Napier’s discovery of logarithms. Besides being a strange key on your scientific calculator, Logarithms (or logs) were very important in the pre-computer math world. Let’s consider base 10 logs. If you square 10 (that is, take 10 to the second power) you get 100. So the log of 100 is 2. If you raise 10 to the fifth power you get 100000 so the log of 100000 is 5. The numbers don’t have to be integers. So the log of 200, for example is about 2.3.
Table of Logs
If you spent a lot of time calculating, you could create a table of numbers and their logs. The question is: why would you want to? The answer is simple. Suppose you wanted to multiply two numbers. I’ll do 200 and 100 first, even though they are easy enough to multiply without any tricks. If you don’t use any tricks (or heuristics, if you prefer) then you’d write 200 over 100 and then multiply each digit. With logarithms, however, we can do it much more easily. The log of 200 is 2.301 and the log of 100 is 2. So the log of result we want is the sum of the logarithms (2.301+2=4.301). if you compute 10 to 4.3 power, you’ll see it isn’t quite the right answer (19998.6) because I rounded the log of 200, but it is pretty close. Clearly, the more digits you have in your log table, the better.
That seems like a dumb example, but if you wanted to multiply 7329 and 8115, it is a lot easier if you knew that the logs were 3.8650 and 3.9093, respectively. Adding those gives you 7.7743 which is the log of the result. Just look that up in your handy log table to find out the answer is 59470282 (well, it is actually 59474835, but, again, pretty close).
Movable Tables
What’s this have to do with a slide rule? The slide rule is effectively a log table on two pieces of wood, plastic, or metal (bamboo rules were especially prized because they were self-lubricating, felt good to handle, and were very stable). The marks are put down based on the log of the number, but the marks are labeled by the actual numbers. So the distance between 0 and 1, for example, is much greater than the distance between 8 and 9.
 Let’s consider a very simple example: 2 times 3. If you move the slide (the C scale; see right) so the one is over the 2 on the fixed scale (the D scale), you can then count over to the 3 mark on the sliding part. This is the same as adding log(2) and log(3). Now you only have to look down from the 3 mark to the fixed scale to see the answer (6). This is very easy to understand when you have one in your hand. For those that don’t, try this web simulator. A screenshot of the calculation is found at the bottom of this section.
Let’s consider a very simple example: 2 times 3. If you move the slide (the C scale; see right) so the one is over the 2 on the fixed scale (the D scale), you can then count over to the 3 mark on the sliding part. This is the same as adding log(2) and log(3). Now you only have to look down from the 3 mark to the fixed scale to see the answer (6). This is very easy to understand when you have one in your hand. For those that don’t, try this web simulator. A screenshot of the calculation is found at the bottom of this section.
In some cases, moving the slide may put the answer “off the scale.” In that case, you can use the right hand side of the sliding scale (which is often marked 1, but really represents 10). Then you move to the right and remember to scale the result up by 10 (some rules offer other ways to deal with this, too).
If you want to do bigger numbers, you first scale them down and then mentally scale the result back up. So computing 20 times 30 or 2 times 30 is the exact same procedure, but you know you have to scale the answer up by the number of places you shifted. Same thing if you wanted to do, say, 25 times 3.1. You’d actually multiply 2.5 times 3.1 and then scale the result.
Division and Other Operations
Division works almost the same way but relies on subtraction. If you line up the 3 on the sliding part with the 6 on the fixed part, you can look under the 1 on the sliding part and see the answer is 2. To help you read the numbers accurately, there is a little plastic cursor with a hairline for lining up the numbers. Some rules even had a tiny magnifying bubble there to help you read more accurately.
Fancy rules will have other scales. For example, the A scale does squares and square roots and trig scales were also common. If you want a visual demo of how it works, check out the video below.
Getting the Right Answer
Unlike a calculator, a slide rule does require you to have some idea what the answer is (in scale) to interpret the result. It also relies on you being able to eyeball the difference between, say, 7.3, 7.35 and 7.351. That’s why bigger is better.
A “normal” slide rule is usually about 10 inches long. There were pocket rules that were shorter and even one on a tie tac that wasn’t very practical to use. On the other side of the spectrum were giant slide rules meant for use in a classroom (some were 7 feet long). For very precise work, engineers would use rules shaped like a cylinder. With the scales wrapped around the circumference of the cylinder, your desk could hold the equivalent of a 30 foot slide rule.
 However, there was another odd sort of slide rule that was the same a 66 inch rule, but would fit in your pocket: the Otis King (also known as the Geniac; see picture to the left). At first glance, you’d think this slide rule was a telescope. But it is actually a slide rule with the scales wrapped in a spiral around the instrument. By telescoping the scales out, it was–in theory–possible to read more digits off than a normal slide rule. However, due to inaccuracy in the scale markings, it wasn’t always as accurate as it should have been.
However, there was another odd sort of slide rule that was the same a 66 inch rule, but would fit in your pocket: the Otis King (also known as the Geniac; see picture to the left). At first glance, you’d think this slide rule was a telescope. But it is actually a slide rule with the scales wrapped in a spiral around the instrument. By telescoping the scales out, it was–in theory–possible to read more digits off than a normal slide rule. However, due to inaccuracy in the scale markings, it wasn’t always as accurate as it should have been.
Where to Find Out More
The Oughtred Society is a wealth of information about slide rules, including tutorials, history, and pictures of common and rare instruments and links to other interesting places.
If you’d rather be hands on with virtual slide rules, there are plenty of those and a search for “slide rule emulator” or “slide rule simulator” will turn up lots of sites like this one. Faber-Castell made a lot of European slide rules and they have an interesting page about their history in the slide rule market.
Where to Find Collectible Slide Rules
You’d think it would be hard to collect slide rules, but it is actually fairly easy and can be inexpensive. The trick is that they were so widespread and disappeared so abruptly that there are plenty of used and new old stock rules out there if you can find them. Faber-Castell even mentions they still have some that they will sell you at the bottom of their history page.
eBay is a prime source for slide rules (a quick search showed over 3000 listing related to slide rules). You may find you can get them cheaper by combing local antique stores. Often people don’t really know what they are and are glad to get rid of them. Also, if people find out you are a collector, they will often give you slide rules that belonged to some long gone relative and will be glad that someone will have it who will appreciate it and take care of it. I have several of these.
If you do want to buy a rule, there are a few things to look for. First, make sure it has the cursor and that it isn’t fogged up. Repairing or replacing a cursor is often a big ordeal. Watch out for corrosion or color leaching from a leather holster. These rules can often be saved, but it is a lot of work. The Sphere site has some good model-specific instructions for cleaning different rules, as does another Canadian site. Irregular gaps between the slide and the fixed part can spell trouble and if you can handle the slide rule, make sure the slide and cursor both operate. It is also useful to see if the rule is warped, which is probably impossible to fix adequately.
Keep Sliding
If you do acquire a slide rule, you’ll need to remember a few things about taking care of it. Although bamboo is self-lubricating, other slide rules may need a little help sliding. Pledge (the furniture polish spray) works well on wood rules. Petroleum jelly was what most people used on metal rules. A little bit goes a long way. It is important to keep a slide rule clean so that grime doesn’t get under the cursor where it is more difficult to clean and interferes with the operation of the rule.
Be careful putting your slide rule in direct sunlight. Depending on the type of rule you have, you should be careful using water, soaps, or solvents that might damage it. Be sure to read the cleaning sites I mentioned above and try to test things a bit if you can find an inconspicuous spot before you start washing the whole rule down with something.
I collect a lot of old gear and, to me, these were the computers of their day. Not everyone wants to learn Morse code, or know how to bias a tube, or be proficient at driving a stick shift. But a lot of people enjoy keeping this kind of old knowledge alive and–you never know–when the apocalypse occurs, the slide rule jockeys left might be the best computers you have for a while.















“the distance between 0 and 1″…. Hmmm, there is no 0 on a slide rule ;)
well, there would be a zero on an infinitely long slide rule ;)
Maybe someone can do a write up on the E-6b flight computer.
Thanks. That was just what I was about to say. I still use these flight computers both for flying and in everyday life (bezel on my wrist watch). There is just no easier way to find out things like “it took me 12 mins to fly 17 miles, I need to go 102 miles, how long will that take”.
Line up 12 with 17 and read off from 10.2 ish?
Great suggestion, thanks! If you have more we’d love to hear them in the tips line.
Oh boy! I haven’t used my E-6B in flight since getting Forefight. I should whip out that bad boy one of these days and do a cross country flight with it.
I hated the e6b when I had to use it, but I loved it as soon as no one forced me to use it.
I have a slide rule bouncing around on my desk mostly as a desk toy. But a few weeks ago, I needed to know the cube root of some number or other and saw the slide rule before I managed to find the calculator. (Yes it’s a messy desk.) It did the job.
The history of calculating devices at the beginning of this article is great, but overlooks my favorite (really only for the name, which makes a great old-timey exclaimation) Napier’s Bones – https://en.wikipedia.org/wiki/Napier%27s_bones
+1
(You beat me to it…)
A circular slide rule got me through my college years. It was easier to carry than a straight one, since it fit right on top of my books. I didn’t see anyone else using a circular one.
Dr. Strangelove
Somewhere I have a link to a printable circular slide rule that fits in a CD case…
We’ll be discussing log tables next. At least I still have my slide rule from school, but I think I used log tables more. By the time I started university, Uncle Clive Sinclair had come out with the Sinclair Cambridge and the Sinclair Scientific calculators. Log tables were still more accurate ;-)
The day my calculator batteries gave out just as I was starting a physics exam I went out and bought a reference with a set of log tables in the back. I think I still have it somewhere with my old textbooks.
Still, the Sinclair scientific was genius, as they crammed it into the same ROM as a four function TI calculator:
http://files.righto.com/calculator/sinclair_scientific_simulator.html
It was also the first calculator my family bought, in the mid-1970s. However, being 7 years old I hadn’t heard of RPN and so I couldn’t figure out how to do calculations with it. So we took it back and swapped it for a four-function Commodore calculator. What a missed opportunity!
I have a “Thachers Calculating Instrument” that belonged to my uncle, who was a professor of engineering and metallurgy.
It’s in great shape, and I am toying with the idea about selling it on ebay.
If it matters, the CSG-1 and CSG-2 are Jeppesen’s designations for the small and large E6B originally developed by Dalton and Weems and still used in flight schools everywhere. A more compact device (though less intuitive for crosswind calculations) is the CR3 that can be a lot of fun to give to students who forget their calculators in an exam.
I still have the slide rule I used in high school, only getting an electronic calculator my Senior year. Using a slide rule is faster than pushing buttons, schools even had speed competitions.
When it’s within reach I find it a lot quicker than calc.exe, Google or my phone just by the number of steps it takes (as long as it’s just multiplication).
Have several slide rulers, but more scientific and graphic calculators I can recommend installing a nice calculator app on your mobiles/smartphones. I prefer Wabbitemu with Ti83+ rom since Im used to my real Ti83+, and really handy to have with you most of the time on the phone. Quite the irony to use a powerfull computor with HD touchscreen to emulate a slow 25 year old computor with a bad screen.
Slightly off topic, sorry, but has anyone else noticed that the high end calculators (TI, HP, etc.) are worse, yet they seem to be the only device not following Moore’s Law? I use a ~30 year old HP 11C because the buttons are far better than anything made since, how many people still use RPN?
I am probably a bit weird in that I have a home made DIY circular slide rule in a leg pocket and a HP 35s (not HP-35) in my backpack and another one close to my desk at home.
The circular slide rule have gotten a bit worn though (again). I need to come up with something more robust than discs of laminated heavy office paper (I think I used 120 grams/square meter paper and 120 micrometer laminate for heat laminating office machines). Do a Google image search for “johan g diy circular slide rule” to see it. ;-)
A key programmable scientific RPN calculator really makes a difference when I want to work out some algorithm or want to do some repetitive calculations without starting up the computer. Best way to document the programs? Forth stack diagrams!
I have 10 year old Casio that is ok but the buttons really don’t feel that robust. The TI-85 I used in high school however was built like a tank, I’m surprised they don’t ban them from schools as a potential weapon.
Once you go RPN, you never go back.
Ah, I still remember my first time… Summer of freshman year of college, had a job working in a plywood plant. Blew over a weeks pay on a HP-41CX. Never looked back. Still remember my first program (it actually ties into the slide rule article). I’d read an article about one of the cable scrambling systems that stated there were 480! (factorial) possible combinations of scanlines in that particular scheme. Wrote a loop to estimate that value (by summing logarithms, of course… see it ties in). Yeah, pretty sad. But I can make it sadder. A few years later the 48SX came out. I was co-oping at the time and was able to attend a presentation held at the HP office in Huntsville, AL. I went with another HP enthusiast. We sat through the demo (they had modified a 48 display so it could sit on an overhead projector, really neat) and fought to urge to go to the back of the room where the local campus bookstore had set up a table with boxes of the calculators and a credit card machine. Later that night, I sat down and tried to figure if I could afford to get one by the end of the co-op term. After plugging in numbers for a while I finally looked at the display and noticed that the total didn’t look right. After pushing some buttons I realized that the 9 key had become flaky. I took it as a Sign. The next morning I went in to work and ran into the guy I went to the demo with. He just looked at me. “You’re getting one, right?” “Yup.” “…I’ll drive.”
I got into RPN in 1986, with the HP-41cx. I still use it every day, and a few programs I use all the time have been in it continuously for over 25 years, never having to re-load them. There are a few engineers who are fanatical about this machine who are still introducing new modules for it. One also sells the 41CL transplant board that’s 50 times the speed of the original and comes with over 230 modules pre-loaded.
Please mention that website much requires you supply a valid hp room!
A friend of mine in college had a Curta:
https://en.wikipedia.org/wiki/Curta
It was, he said, more accurate than a slide rule. I think it was slower, also. He wouldn’t let me use it.
I sodl one of those on ebay a couple of years ago. It made £400 or so, I was gobsmacked.
Whenever I design the geometry for a new recumbent bike project, I use one of my slide rules strictly for the fun of it. Keeps me in practice just in case there is a zombie apocalypse. As always, the hardest part is remembering where to put the decimal point.
I used an expensive bamboo slide rule once to fix a table that was a bit wobbly. I moved the table around a bit and found which leg was the shorter one and wedged the slide rule under it. Hasn’t wobbled since!
Check shopgoodwill.com…they usually have a couple, and your money goes to a good cause.
Some of your money goes to a good cause, the rest goes to the CEOs six-figure salary.
who then promptly goes and buys Goodwill furniture for his home, if the story I read in the local newspaper is true.
Studying engineering in the age of a slide rule, as I did, had a nice benefit. Since accuracy degrades if you must make multiple calculations, test results only had to be accurate to two digits (i.e. 3.4).
The downside is that slide rules don’t manage your exponents well, leaving the possibility hat the answer is 0.34, 3.4 or 34. You found what was correct by doing rough calculations in your head. Multiplying 2.31 by 38.5 became multiply 2 by 40, so the result needed to be roughly 80. My calculator says it is 88.935. A small slide rule would probably get you close to 89 for the answer, so 89 would be what you would put down.
On the other hand, there were ways to get highly accurate results. I’ve been told that the lunar missions (i.e. Apollo 13) used very large slide rules for calculations. The result would be available in seconds. It’d take too long too program a mainframe.
That’s exactly why I keep an abacus around. You never know when you’re going to need precision out to a few beads.
Lol!
“You found what was correct by doing rough calculations in your head”
Which is exactly what you *should* be doing. Kids nowadays.
// uphill both ways
One should do that even with a calculator, because wildly differing answers alert you to the fact that you made an entry mistake.
LOL, When I was in 6th grade I was told to leave the class cause I was cheating using a slide rule. Looking back maybe I was but hey !
I used one in Jr Hi and other kids said I was cheating but the teacher, seeing my engineering bent, not only tolerated it, but seemed to encourage it.
Some old slide rules are worth quite a bit. I got my grandpa’s surveying slide rule and looked it up and they sell for over $400. Yikes…
Faber-Castel is still selling them, brand new, up to 20″ rules. See http://service.de.faber-castell-shop.com/epages/es117781.sf/de_DE/?ViewAction=View&ObjectPath=/Shops/es117781_ServiceParts/Categories/Faber-Castell/FaberCastell_Rechenstaebe&PageSize=50&Page=1 .
I got the last slide rule they passed out in my high school when I started 10th grade. The other kids were so jealous. I still have it in my den. I also have the tie-tac slide rule the author mentioned.
It the tie-clasp one this one? http://wilsonminesco.com/SlideRules/SlideRules.html#Tie
More likely than not dist/ dust and a tool to make scratches in either is likely to survive any apocolypse, the truly prepared will keep brains exercised to to perform calculations with only those two simple tools. :) I’m reminded of a scene in a movie about some early exploring expedition. The navigator of the expedition was pissing and moaning that he couldn’t to his work because hi book of log and other tables was lost in a boat capsize in a river. The captain said tough shit do your job by making the calculations with out aids.
I have the engineering standard, the Post Versalog made of bamboo, with the leather belt case and manual. I picked it all up via ebay about 15 or 20 years ago. I went to high school in the 70s right at the time electronic calculators first became widely available. I remember seeing seniors walking around school with slide rules hanging from their belts. I bought a calculator that had a green fluorescent display and a pi key. I went through a series of calculators over the ensuing years, but my favorite of all is my HP-11C, still working perfectly, and has only had the batteries replaced twice in 20+ years of daily use. I bought an HP48GX and the keyboard lasted about 1 year. One of these days I’m going to get a Curta.
Rockwell Calculators, they really can’t be beat,
they’ve got big green numbers and little rubber feet!
The foundations of slide rules are discussed in a nice whitepaper I found (pdf!): https://cseweb.ucsd.edu/~pasquale/Papers/IM11.pdf
The main takeaway is that you can have a function of four variables, set any three and get the fourth. The function needs to be linear, or transformable to linear with respect to three variables. This gives quite remarkable computing power for a device as simple as a slide rule. I designed a special application slide rule based on this, and it is quite handy for its job of instrument setup: https://sites.google.com/site/dmacalculator/
Why a slide rule is better than a PC
http://bilbosrandomthoughts.blogspot.in/2010/04/why-slide-rule-is-better-than-pc.html
1. A Slide Rule doesn’t shut down abruptly when it gets too hot.
2. One hundred people all using Slide Rules and Paper Pads do not start wailing and screaming due to a single-point failure.
3. A Slide Rule doesn’t smoke whenever the power supply hiccups.
4. A Slide Rule doesn’t care if you eat or drink while using it.
5. You can spill coffee or a soft drink on a Slide Rule and keep on computing.
6. A Slide Rule never sends you snarky system messages about upgrades, reboots, and damaged files.
7. A Slide Rule and Paper Pad fit in a briefcase with space left over for lunch or a change of underwear.
8. You don’t get junk mail offering pricey upgrades for your Slide Rule that fix current old errors while introducing new ones.
9. A Slide Rule doesn’t need scheduled hardware maintenance, an IT staff, and a 24/7 help desk outsourced to a team of geeks in Carjackistan who barely speak English.
10. A Paper Pad supports text and graphics images easily, and can be easily upgraded from monochrome to color.
11. Slide Rules are designed to a standardized, open architecture.
12. You can use a Slide Rule to hit the obnoxious person in the next cubicle.
13. Nobody can steal your identity by hacking your Slide Rule.
14. You can upgrade your memory without limits by simply adding additional Paper Pads. No need to reconfigure anything, change any settings, or do any backups. “Backing up your data” consists of putting the old Paper Pad away in a drawer.
15. Nobody will make you feel bad by introducing a smaller, faster, cheaper Slide Rule next month.
Could a digital slide rule be made from some digital calipers?
No, but just for the fun of it, I had considered making a hexadecimal slide rule.
Yes, digital calipers could be used, if they could be made to read out the log value of the distance. I would not be suprised it that could be done…
When my son went to Field Artillery school (about 6 years ago now), they issued him slide rules for calculating artillery fire. Because you need to know how to do it by hand when your big fancy artillery computer stops working.
I have 2 slide rules, and I still use my HP-11C calculator.
I have a very small slide rule keychain that has year, month, day and name of the weekdays as parameters. Pretty cute thing.
Not that this thread needs more comments, but: A slide rule is really only useful for doing engineering homework and taking tests. In the real world one needs more than 3 significant digits, as most calculations involve taking a difference of prior calculations which makes the dubious 3rd significant digit even more dubious. The HP35 RPN calculator was the killer app that blew away the slide rule. For $5 you can download an HP42s app from the Apple store which is as good a calculator as was ever made. If you need more computational power, you probably should be using a spreadsheet. If the price is too high, I believe there is a free version also.
The Empire State building, Golden Gate bridge, aircraft and early spacecraft, even the F-16, were designed using slide rules; so they definitely were a serious tool engineers depended on heavily. I used a slide rule a lot in electronic circuit design until calculators really took hold and the price of the ones that could do at least the trig and log functions came down within reach of the common man. Tolerances of resistors used in common products was 5%, and capacitors were usually 10% or 20%. Transistors’ gains, breakdown voltages, etc. had ranges of around 2:1. So yes a slide rule’s accuracy and precision were plenty more than enough for real work. The 3rd digit should not be dubious at all with a well made 10″ rule and decent vision (in fact, checking with my calculator, I find I can nearly always get the 3rd digit correct with my 4″ rule); and chained calculations’ errors should be some plus and some minus, mostly canceling out.
Curta’s (https://en.wikipedia.org/wiki/Curta) were the original personal computer. The slide-rule was the faster approximation to computing.
The video says slide rules were used until 1972 when the HP-35 calculator came out. Actually, the HP-35’s price tag put it out of reach of most of those who would use a slide rule. I remember calculator ads through the 1970’s touting “slide-rule calculator!” meaning it had all the functions of a slide rule, which they usually did not.
The 3rd sig fig was not a guess at all, to someone who was skilled at using the slide rule. In multiplication and division, I can always get the 3rd sig fig off by no more than 1 even on my 4″ slide rule, and I can quite often get the 4th sig fig on my 10″ rules. In chained calculations, the errors will be random, not always high or always low, so they tend to cancel, not accumulate. The man in the video obviously did not know how to quickly micro-adjust the settings by rolling his fingers, so he got very coarse settings. In common log (ie, base e) operations, it is not uncommon to get five digits. In electronics (my field) though, most parts’ tolerance is between 1% and 10%, so three digits is usually more than enough in circuit design.
In high-school physics in 1977, I was the only one in the class still using a slide rule. One of the problems we had to solve was the orbit time of a satellite orbiting the earth. The others thought slide rules were for hardly more than an estimate, so they were surprised when my answer was only four seconds different from the teacher’s which he got with his calculator.
If you use the slide rule all the time, it is not really any slower than a calculator. It adds the benefit of sharpening your mind (which is why I still go back to it once in a while), and helps understand number relations better. An engineer I was working with a few years ago commented after I quickly did several logarithms in my head in the course of our conversation about the product design, “How do you do that?!” I replied, “You’re just a little too young to have used a slide rule, aren’t you?”
My slide rules, and a lot of slide-rule links, are at http://wilsonminesco.com/SlideRules/SlideRules.html
The early programmable and scientific calculators were horrible power hogs. Users were terrified at the thought of losing power in the middle of an exam. They also lost their program contents. At the university, I obtained an HP25C, the C for Continuous memory, it retained the program contents after it lost power.
I got my first calculator in ’76 or ’77 which had a very short battery life. It had four functions plus square root and percent, and that’s all. I mostly used the slide rule until I decided I needed something programmable to handle iterative operations which would loop thousands of times. The slide rule was not programmable. In Dec ’81, I took the plunge for a programmable, a TI-58c. The earlier version, without the “c,” seemed somewhat pointless because it did not have continuous memory and you could not load programs in from cards like you could the 59, and battery life was only about three hours.
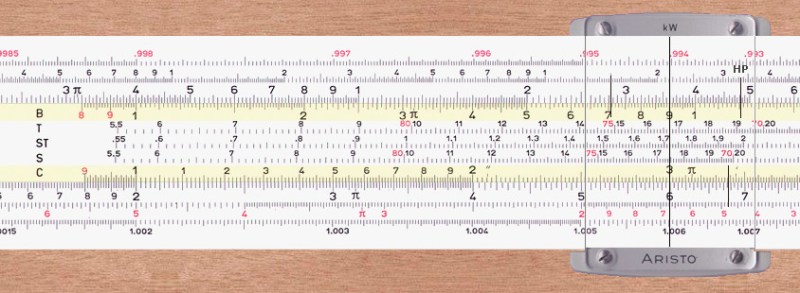
You will notice cryptic marks on the cursor of a slide-rule, and elsewhere, called gauge points. I had a Staedtler slide-rule in the 70s but the instructions didn’t explain those marks.
The center line has kW written on it, that’s kilowatts. There is a short line on the side of the A and B scales that’s HP, so you can do kilowatt to horsepower conversion (1 horsepower = 0.7457 kW). Put the HP line on a 1 on the A-scale and you’ll see the main (kW) line lines up to this value.
Also there are short, unmarked lines to the top left and bottom right of the main line. These are for converting the diameter of a circle to its area and vice-versa. Put the main line on the 2 on the D-scale. The A-scale will land on the tiny tick used to represent the value of pi. A circle’s area is pi times the square of the radius, so a circle with diameter of 2 (radius of 1) has an area of exactly pi. To go in reverse, line up the value for the area on the D-scale, and the bottom right line on the A-scale will give the diameter.
If you are sharp-eyed, you will notice two tiny ticks on the C-scale: ” and ‘. These refer to degrees, minutes (“) and seconds (‘). “(rho)’ at 3438, and (rho)” at 206255, in scale C, give the numbers of minutes and seconds in a radian respectively. These gauge points may be used for finding the values of trigonometrical functions of small angles. For any small angle, say, less than 2°, the sin and the tan may be taken as identical. If we set the r’ mark to the graduation in scale D, representing one-tenth of the number of minutes in the angle, the sin or tan may be read in D under the 1C or l0C.”
One of the beauties of a slide rule that no-one seems to have mentioned is that when using it, you have the scales before your eyes. You can see the relationships between the scales not just the number(s) that you are interested in, whereas with a calculator, you pop in a number or a couple of numbers and out comes a single number. Any relationship between what you put in and what comes out is simply not in view. I used a slide rule regularly to demonstrate this to undergraduates who had relatively poor maths skills but could quite happily ‘use’ an electronic calculator. A slide rule is a little work of visual art that you can learn a lot from simply by just looking at it. While you may admire the design of an electronic calculator, it teaches you little about maths and at best only the answer to a single problem each time you use it.
Howard Speegle of Diva Automation game me his permission to re-tell his story:
With your background, you might enjoy some aspects of my work on the Nimbus weather satellite. It had a 250 mW transmitter and our 85-foot dish with Maser amplifier could achieve autolock at -150 dbm at a range of 3000 miles.
However, the launch vehicle suffered an early burnout and the orbit was degraded, causing the satellite to be lost to the free world for three days. No one at NASA Goddard or the DEW line or any tracking stations around the world were able to locate any evidence that it existed. We scanned the skies continuously in every sort of random and geometric pattern for days, but no cigar.
Finally, I whipped out my trusty circular pocket slide rule…and came up with a reasonable approximation of what the orbit would look like with a 10 second premature shutoff and suggested to my boss that they point the antenna in a certain direction at a certain time.
And lo!, it came to pass. He wanted to know where I had studied astrophysics and I didn’t think he would appreciate knowing about my plastic slide rule so I simply shrugged it off.
A few weeks later, the ham club at the University of Alaska, down the road, timidly asked if we might have any interest in the telemetry tapes they had made of the first pass. A bunch of kids using a hand-pointed chicken wire parabola had made beautiful recordings of the entire pass and subsequent passes. They had outperformed the combined might of NASA and the millions upon millions of dollars of state of the art equipment we had. Kudos to them. I don’t know whether we ever thanked them. Perhaps I should send this story to the university so they can add it to their list of accomplishments.
I recollect struggling with long division and multiplication at junior school. The maths teacher couldn’t understand why my answers were often out by a few decimals. She watched me and realised why… I had been using log tables and then adding / subtracting logs rather than multiply or divide. Errors in the log table book meant my approach didn’t have reliably wrong answers.