We like mechanical calculators like slide rules, but we have to admit that we had not heard of the Ott Derivimeter that [Chris Staecker] shows us in a recent video. As the name implies, the derivimeter finds the derivative of a function. To do that, you have to plot the function on a piece of paper that the meter can measure.
If you forgot calculus or skipped it altogether, the derivative is the rate of change. If you plot, say, your car’s speed vs time, the parts where you accelerate or decelerate will have a larger derivative (either positive or negative, in the decelerate case). If you hold a steady speed, the derivative will be zero.
To use the derivimeter, you sight the curve through the center glass and twist the device so the cursor, which is a lens and mirror system that lets you precisely find a tangent line. You can read the angle and find the true derivative using a table of tangents.
[Chris] has another derivimeter from Gerber. However, he found a different type of derivimeter that uses a prism, and he sure would like to find one of those for his collection.
Calculus is actually useful and not as hard as people think if you get the right explanations. This isn’t exactly a slide rule, but since it is a mechanical math device, we think it counts anyway.

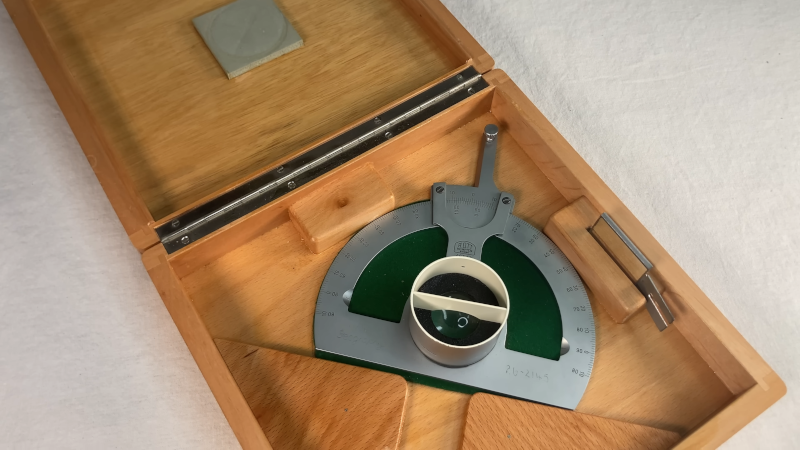














It did however fail to find the correct spelling for “derivatives” 😂
Fixed, thanks!
Perhaps 3D print and laser cut acrylic to make your own prism derivimeter until someone sells you an original.
I wouldn’t say it finds the derivative, I would say it finds the local value of the derivative function. After all you don’t tell it the function is f(x) = 4x^2 – 3x, you plot out some values for it.
I may have forgotten some of my calculus, but I can tell you that f'(x) = 8x – 3. I suppose if you graph out the derivative values using the device, you could figure that out, or something near it.
Your response might be [need voice] teknikally [/nerd voice] correct but that’s the only way in which it’s correct.
By your reasoning a ruler doesn’t give you the dimensions of a thing, it just gives you the specific distance between the two particular points you choose to put it on.
It’s obvious what was meant by ‘giving the derivative’
Nawwwwww. Having an expression for slope of a curve at any point on that curve is way more useful- any freshman calculus course has you solve general solutions algebraically first then “plug and chug” with values to get a desired solution. Its only way later with integrals and differentials that “sketch first estimate answer call it good” becomes viable or more efficient for things that cannot be solved in general.
Except this ignores what devices like rulers are for. Generalized solutions are great, but depending what you are doing they are not necessary.
I was surprised to find my City of birth in a Hackaday video (Where the Ott company resides)
I would like to see how “good” this is compared to just a ruler and pencil, if you have to plot the thing on paper to begin with anyway. Considering that differential calculus is pretty trivial for most uses it’s hard to imagine someone that one couldn’t just do the math or numerical approximation but this device is quick and dirty enough. Beautiful instrument though for sure. Like. Plotting it by hand would take way longer than doing the derivative and having an analytical solution at more than one point. And if the plot is machine plotter or computer made anyway, again, computer numberical approximation would be trivial as well. ??
Well you could watch the video, where he shows that eyeballing the tangent is much less accurate. Anyway, considering they probably weren’t idiots, I suspect the idea was that you probably didn’t have the function solved in the computer just sitting there waiting for you to query it. They’re old tools, you know; for a long time the hard copy was the source of truth and not a digital file in a datacenter on the internet.
How to buy the instrument ?
If you find out, please post.
It is possible to buy the instrument?
I am working on an exhibit about calculus. I would like to purchase an Ott Derivimeter. Any suggestions?
Cliff Landesman